In this article, we will discuss about the subject-matter of advertising and rule of thumb of advertising.
Subject-Matter of Advertising:
We have seen that firms can utilize their market power when making pricing decisions.
Pricing is an important decision for a firm, but most firms with market power have another important decision to make — how much to advertise. Here we will see how firms with market power can make profit-maximising advertising decisions, and how those decisions depend on the demand for the firm’s product.
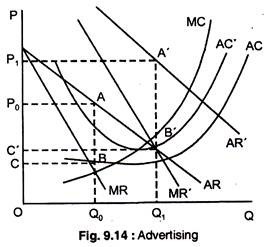
For simplicity, we assume that the firm sets only one price for its product. We also assume that it knows how its quantity demanded depends both on its price P and its advertising expenditure A; that is, it knows Q (P, A). Fig. 9.14 shows the firm’s demand and cost curves with and without advertisement.
ADVERTISEMENTS:
AR and MR are average and marginal revenue curves when it does not advertise, and AC and MC are average and marginal cost curves. It produces a quantity Q0 where MR = MC, and fixes a price P0. Its profit per unit is the difference between P0 and AC, so its total profit π0 is given by the area ABCP0.
Now, suppose, the firm advertises. This causes its demand curve to shift to the right; the new average and marginal revenue curves are given by AR’ and MR’. Advertising is a fixed cost, so the firm’s AC curve rises to AC’. MC remains unchanged with advertising, the firm produces Q1 (where MR’ = MC) and receives a price P1. Its total profit, π1 is now much larger. Advertising is worthwhile because it increases both AC and AR = D.
While Fig. 9.14 shows that the firm is better-off advertising, it does not help us to determine how much advertising the firm should do. The firm must choose its price, P, and advertising expenditure, A, to maximise profit, which is given by π = PQ(P, A) – TC(Q) – A.
Given a price, more advertising will result in more sales, and, hence, more revenue. But what is the firm’s profit-maximising expenditure? Profit-maximising advertising is a point where the MR from an additional advertising, MR’ = MC (full MC of advertising).
ADVERTISEMENTS:
That full MC is the sum of the money spent directly on advertising and the marginal product cost that results from the increased sales that advertising brings about. Thus, the firm should advertise up to the point that MR’ = P dQ/dA = 1 + MC dQ/dA ………(3)
This rule is often ignored by managers, who justify advertising budgets by comparing the expected benefits only with the cost of advertising.
A Rule of Thumb for Advertising:
Equation (3) is sometimes difficult to apply in practice. We saw already that MR = MC implies the following rule of thumb for pricing: (P-MC)/P = –1/Ep, = where, Ep is the price elasticity of demand. We can combine this rule of thumb for pricing with equation (3) to obtain a rule of thumb for advertising. First, rewrite equation (3) as follows: (P – MC) dQ/dA =1
ADVERTISEMENTS:
Now multiply both sides by A/PQ, the advertising-to-sales ratio: = (P/MC)/ [ A/Q. dQ/dA] = A/PQ The term in brackets, (A/Q) (dQ/dA), is the advertising elasticity of demand. We will denote this elasticity by EA. Since P-MC/P = 1/EP. = we can write this equation as:
A/PQ = EA/EP…………………..(4)
This is a rule of thumb for advertising which means that to maximise profit the firm’s advertising-to-sales ratio should be equal to minus the ratio of advertising elasticity to price elasticity of demand. Given information on these two elasticities, the firm can use this rule to check its advertising budget.
The firm should advertise a lot if: (i) demand is very sensitive to advertising (EA is large) or (ii) demand is not very price elastic (Ep is small). While (i) is obvious, why should a firm advertise more when Ep is small? The reason is that a small Ep implies a large mark up of price over A/C, so that the marginal profit from each extra unit sold is high. In this case, if advertising can help sell a few more units, it will be worth its cost.