When not only degrees of risk differ but also expected values of profits from investment projects are different, on what basis the individual or manager will make a choice among the investment projects?
If the manager of a firm is risk neutral or risk seeker he will choose to undertake the investment project whose expected monetary value is positive.
However, if the individual entrepreneur or a manager of the firm is risk-averter, it is not necessary that he will choose the investment project if expected monetary profit is positive. Whether or not he will undertake investment in the project depends on his utility function of money. Let us take an example.
The utility approach to risk measurement we are in a position to explain how a manager faced with investment projects with different expected values and different degrees of risk will choose an investment project. From the viewpoint of utility approach, an individual is risk-averse whose total utility curve of money is concave (i.e. face downward) as shown in Fig. 17.3 in which marginal utility of money for an individual diminishes.
ADVERTISEMENTS:
Problem 1:
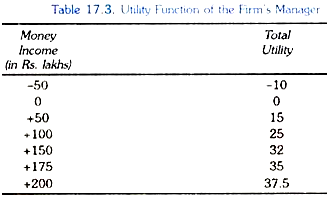
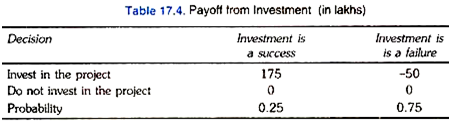
If an individual who is risk averse and therefore his marginal utility of money will diminish as he has more of it. Suppose such a risk averse entrepreneur or manager has to decide whether or not to undertake investment in a project. It is given that if it proves success, it will yield profits equal to 175 lakhs and the probability of success is 0.25 and if it proves to be a failure he will lose Rs. 50. lakhs and the probability of failure is 75 per cent. Utility function of firm’s manager is given in Table 17.3. in the form of a payoff matrix. Whether a manager will decide to invest in the project?
In order to assess whether a manager will decide to invest in the project, we calculate below the expected monetary value of the profits of the investment.
Expected monetary value of profits = 175 x 0.25 + (- 50 x 0.75)
= 43.75 – 37.5 = 6.25
Thus expected monetary value of profits is positive, a risk neutral and risk-seeker will decide in favour of undertaking investment in the project. Now whether a risk averse manager will undertake investment in this project. For this we require utility function of the firm’s manager which is given above in Table 17.3. It will be seen from the total utility function of Table 17.3 that marginal utility of money income of the firm’s manager is declining and that means he is risk averter. Now we can calculate the expected utility of the manager from investing in the project.
Thus, E (U) = U(175) x 0.25 + U (-50) x 0.75.
ADVERTISEMENTS:
It will be seen from Table 17.3 that utility of Rs. 175 laks of the manager is 35 and the utility of loss of Rs. 50 lakh (i.e. – 50 laks) for the manager is -10.
Putting them in the expression for expected utility we have:
E (U) = 0.35 x 0.25 + (-10) x 0.75
= 0.08 – 7.5
= -7.42
Since the expected utility from the project is negative, a risk-averse manager will not invest in the project. Thus even though expected monetary gain for the manager from the investment project is positive, his expected utility from it is negative. It may however be noted that utility functions of different risk-averse managers are quite different and marginal utility of money income for different managers diminish at different rates.
It is therefore possible that for some risk-averse managers the utility function of money may be such that expected utility from the above investment project may turn out to the positive and therefore he will decide to undertake investment in the project.