Derived-Demand Elasticity (or Elasticity of Factor Demand)

Derived-Demand Elasticity (or Elasticity of Factor Demand)!

The observation that the producer demands factor services not for direct consumption for himself but as a means by which he hopes to make a profit. With them he can produce something the consumers are willing to buy and are ready to pay for.

Since it is the consumer’s wish to buy products that causes producers to buy factors of production, the producer’s demand for input is said to be a derived demand (derived from the consumer’s demand for the product). The input-price elasticity of the producer’s demand for factors may, therefore, be referred to as the elasticity of derived demand.

Let us assume to start with that factor services in the productive process are combined in fixed proportions. Marshall’s classic example is that of knife blades and knife handles, which are combined in a ratio of one to one to make knives. According to Marshall, there are four determinants of derived demand elasticity.

The first is the relative ease with which the factor of production in question may be used in other processes, or that with which other factor services (not presently being used in the productive process) may be substituted for the factor under consideration. This is actually a question of the relative difficulty of changing the production function.

The example that Marshall used in explaining the first determinant was that of the services of plasterers.

If the wages of plasterers were to rise, one of the factors determining the relative decrease in the quantity demanded of their services would be the feasibility of altering the productive process so as to use the services of bricklayers, instead. The more feasible the change, the more elastic the demand for the services of plasterers.

The second proximate determinant of derived-demand elasticity seems to be the product (price) demand elasticity. A change in input price will change product price and output. The greater the change in output, the greater the change in the quantity demanded of the input.

The elasticity of derived demand, therefore, tends to be greater if product-demand elasticity is greater, and less if product (price) elasticity of demand is less.

The third major determinant of derived- demand elasticity is the proportion of total cost accounted for by the factor under consideration. This is sometimes called “the importance of being unimportant”. The greater the proportion of the cost of the product attributable to the factor service in question, the greater will be the elasticity of derived demand for it.

Thus, if the price of a factor that is a major cost component increases there will surely be a relatively large drop in the quantity demanded of this factor. If the cost of the particular factor is a small element in the cost of producing the product, there is likely to be a relatively small decrease in the quantity demanded of this factor in the event of a rise in its price. Hence, the importance of being unimportant.

The final determinant of the derived-demand elasticity of a given factor service is the price elasticity of supply of other factors (currently being used in the production process). If purchase of less of these factors causes a fall in their prices, the savings accruing to the firm from purchasing less of them can be used to offset the reduction in the quantity demanded of a given factor service, the price of which has increased.

Thus, if the price of one factor increases, the quantity demanded of this input will fall less if decreases in output cause other factor prices to fall, than if these prices are not affected. The derived demand elasticity for a given input will thus be greater, the larger is the elasticity of supply of other factors.

If we now relax the assumption that factors must be combined in fixed proportions, the same analysis will apply so long as the elasticity of substitution is less than product-demand elasticity.

A fall in the price of an input will now cause an increase in quantity demanded because of the output expansion effect of the price change and its input substitution effect. The relative ease with which this factor is substituted for others will depend upon the elasticity of substitution.

So long as the elasticity of substitution is less than product-demand elasticity, the elasticity of derived demand is simply increased by the fact that this factor can be substituted for other factors and vice versa. Given the inequality, the greater the elasticity of substitution, the larger the derived-demand elasticity.

A Mathematical Note on Derived-Demand Elasticity:

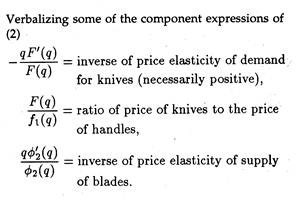
Following Alfred Marshall, suppose factors of production are combined in fixed proportions to produce a product (one knife handle and one blade to produce one knife), let

q = output of knives

F(q) = demand for knives

(q) = supply of blades

m = price elasticity of the demand for handles.

Now, since the price that the firm is willing to pay for handles equals the price it receives for knives minus the price it must pay for blades, we get

(1) f₁(q) = F(q) – ɸ₂(q) = demand for handles.

The price elasticity of demand for handles is, therefore,