This article will help you to learn about the difference between Marginal Efficiency of Capital (MEC) and Marginal Efficiency of Investment (MEI).
Difference between Marginal Efficiency of Capital and Marginal Efficiency of Investment
Keynes used the term ‘marginal efficiency of capital’ to refer to the unique rate of discount which would make the present value of the expected net returns from a capital asset just equal to its supply price when there is no rise in the supply price of the asset.
The term originates with Keynes and is sometimes known incorrectly as the internal rate of return. This latter concept is distinct in that it takes specific account of the fact that the supply price of capital assets will rise in the short run as all firms simultaneously seek to increase the size of their capital stock.
K. Boulding used the term ‘marginal efficiency of investment’ to refer to that rate of discount which would make the present value of the expected net returns from the capital asset just equal to its supply price in the presence of rise in its price even in the short run.
ADVERTISEMENTS:
In the real commercial world a business firm’s decision regarding investment in new physical capital, such as machinery, transport equipment, factories, shores, warehouses, etc. depend on whether the expected rate of return from new investment is greater or less than the market rate interest.
The market rate of interest here refers to the rate that has to be paid on the funds that need to be borrowed to acquire these assets. The assets should be acquired or the projects should be undertaken if the expected rate of return exceeds the market rate of interest.
Profitable investment decisions always involve comparisons of alternative rates of return. Whether the funds are available within the firm from undistributed profits or have to be borrowed makes no difference. Let r denote the rate of interest or yield on existing assets.
This is both the cost of borrowing funds from the external market and the return from leading a firm’s surplus capital at the market rate of interest. The rate of return on new investment, or the marginal efficiency of capital, is denoted as e. It is interpreted as the ‘expected rate of return over cost’ on the new investment.
ADVERTISEMENTS:
The crucial point of distinction between the rate of return on existing assets r and the expected rate of return over cost on new physical capital e. In other words, e may be interpreted as an internal rate of return to the firm, whereas e is the external rate of return (i.e., the rate at which the firm can lend its surplus money at the market rate of interest).
A simple example will make the concept of MEC clear. Suppose, a firm is trying to decide whether to install a machine whose life is 1 year. It has no scrap value after one year. Suppose also that, after deducting all costs except interest and the cost of the machine, there is a surplus of Rs 1,200 left at the end of the year.
Out of this gross return (profit) a sum of Rs 1,000 is required to cover (repay) the cost of the machine. Thus, the net return (i.e., the rate of return over cost) is Rs 200 which is 20% of the original investment of Rs 1,000. We have calculated this return by equating the cost of the machine, C, with the gross return on the machine, R, discounted by the rate of return, e.
The formula used for the purpose is simple this: C = R/ (1 + e) or Rs 1,000 = 1,200/ (1 + e), so that e = 0.20 or 20%. Thus, if we know the original cost of the machine and if we have an estimate of the expected gross return from it, we can calculate the MEC or the rate of return over cost of the machine.
ADVERTISEMENTS:
Now, consider a situation which is slightly more complicated. Suppose the cash flow or earning from a machine is distributed over a number of years. We also assume that it has a scrap value at the end of its economic life. Let R1, R2,…, Rn represent the cash flow from a new machine in years 1, 2,…, n, respectively.
Let K be the scrap value of the machine at the end if its useful life and let C denote the original cost.
Now, by equating the cost of the machine with the sum of the gross returns plus the scap value, all discounted by the rate of return of the machine, we have:
C = R1 / (1 + e) + R2 / (1 + e)2 + . . . + Rn / (1 + e)n + K/ (1 + e)n … (1)
Thus, if we can estimate C, K and R we can solve for e from equation (1). Here, the decision to make investment involves a comparison of the MEC with the market rate of interest. If the MEC is 10% and the rate of interest is 7%, the purchase of the machine is considered worthwhile.
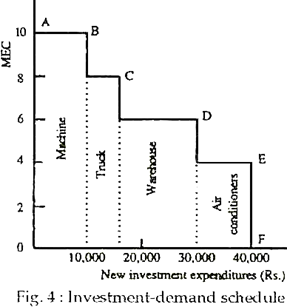
Fig. 4 illustrates the investment-demand schedule of a hypothetical firm. Here, four important projects have been ranked in order of decreasing profitability. The most profitable project here is purchase of a machine which is supposed to produce a return of 10% and whose cost is Rs 10,000. The next best project is a new truck that costs Rs. 5,000. Its MEC is 8%.
The third project involves expanding the warehouse capacity at a cost of Rs. 15,000 to gain an expected return of 6%. The last project in the firm’s portfolio is the installation of an air-conditioner which, by improving morale and raising labour productivity, is expected to yield 4% return.
The solid line ABCDEF in Fig. 4 is the firm’s investment-demand schedule. If the rate of interest is 5%, the firm would drop the fourth project (i.e., purchasing the air-conditioner) and spend Rs. 30,000 on the first three projects (Rs. 10,000 on machine + Rs. 5,000 on truck + Rs. 15,000 on warehouse expansion).
ADVERTISEMENTS:
If, however, the rate of interest is 7%, the idea of expanding the warehouse would be dropped, in which case the firm would spend Rs. 15,000 on capital expansion. Thus, it is quite clear that, for any individual firm the lower the rate of interest, the larger the volume of investment.
The MEC schedule of an individual firm moves along the steps outlined in Fig. 4. By adding up, horizontally, the MEC schedules of all firms in the economy we would arrive at a downward sloping aggregate MEC schedule.
It may apparently seem that such an aggregate of MEC schedule represents the investment-demand schedule for the economy as a whole. But this is not true. It is so because what is true of an individual firm is not true of the economy as a whole.
If all firms attempt to invest more in the event of a fall in the market rate of interest the market price of capital goods will rise. This, in its turn, will lead to a fall in the MEC on all projects for all firms. This very fact that led some economists to draw a distinction between MEC and the marginal efficiency of investment (MEI).
ADVERTISEMENTS:
The latter concept has been developed by K. Boulding and G. Ackley to explain the effect of changes in the prices of capital goods and show the relationship between the rate of interest and the economy’s level of investment on the basis of such changes.
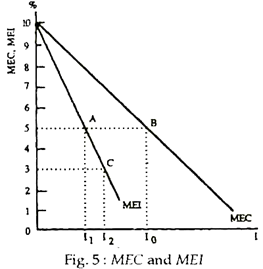
The distinction is brought into focus in Fig. 5. Here, we assume that the market rate of interest is 10%. This very rate makes it worthwhile to replace worn out capital. But, it does not warrant any net (new) investment in plant, equipment and machinery (or any additions to the stock of capital).
We finally assume that the demand for capital goods is not volatile so as to cause any fluctuation in the market price of capital goods. If the market price of capital goods remains unchanged the MEC and ME1 schedules intersect at 10% rate of interest (as shown in Fig. 5).
ADVERTISEMENTS:
Now, suppose, the rate of interest drops to 5%. This will induce each firm to expand its stock of capital. Each will plan for capital expansion such that no one else wished to add to capacity. Thus, all firms taken together would be desirous of spending I0 on new capital goods (as is shown by point B on the MEC schedule). However, when all firms demand more capital goods, the price of such goods will increase.
This, in its turn, will lead to a fall in the MEC of all firms for all their projects. Thus, actual investment will be l1 instead of l0, as is shown by point A on the MEI schedule. Thus, the difference between the MEC and MEI makes all the difference between desired investment (I1) and actual investment (I0) at a particular rate of interest (5%).
Thus, it is clear that the MEC schedule adequately represents the economy’s investment-demand schedule. Like MEC, the MEI schedule is also negatively sloped. But, it is steeper (less elastic) than the MEC schedule. In any case, investment is a function of the market rate of interest. It is inversely related to r.
So, the investment demand schedule may be expressed as:
I = f (r), with ΔI/Δr < 0
In Fig.5, we also observe that if the rate of interest falls from 5% to 3%, the volume of investment rises from I1 to I2.