Models of Economic Growth (With Diagram) | Macroeconomics

In this article, we discuss some basic models of economic growth which lay the foundation for any comprehensive study of the process of economic development. The aggregate production function lies at the heart of every model of economic growth. It is also an extension of the micro-economic production function’ at the national or economy wide level.

The Aggregate Production Function:

Aggregate production function describes the relationship of the size of an economy’s la­bour force and its capital stock with the level of that country’s GNP. If measures the value of output or national product, given the value of the aggregate capital stock and labour force.

Natural resources, such as land, are sometimes incorporated as a third factor, but most often are subsumed as part of the capital stock. The aggregate production function tells us about how capital and labour contribute to growth.

The Basic Growth Model:

Here we present a basic framework to explain the process of modern economic growth. The framework is based on five equations as presented here.

1. An Aggregate Production Function Equation:

The general level production function, i.e., production function for the economy as a whole, is written as,

Y-f (K,L) … (i)

where Y is total output (and, therefore, national income), K is the capital stock and L is the labour supply. Thus, aggregate output is a function of the total stock of capital and the labour force. Output expands with the growth of labour force and accumulation of physical capital. Different growth models, developed from time to time, seek to explain how much output expands in response to changes in K and L.

In this simple framework, economic growth occurs by increasing either the capital stock (through new investment in factories, machinery, equipment, roads, and other infrastructure), the size of the labour force, or both. The remaining four equations of the model describe how K and L increase over time.

2. The Saving Equation:

Total saving is calculated by assuming that saving is a fixed proportion of income:

S = s x Y … (2)

where S is total saving, and s is the saving rate, called the average propensity to save (APS).

3. The Relation between Saving and Investment:

In a closed economy without foreign trade or foreign borrowing, total saving (S) is equal to total investment (I). The reason is that all domestically produced goods and services are used for either current consumption or investment, while all household income must be either con­sumed or saved.

The relationship is expressed as:

S = I … (3)

4. Change in Capital Stock over Time:

Changes in capital stock (K) over time are determined by two factors- new investment (which adds to the capital stock) and depreciation (which slowly erodes the value of existing capital stock over time).

So change in capital stock (AK) is determined as:

∆K = I-(dK) … (4)

where d is the rate of depreciation, I is the increase in capital stock every year by the amount of new investment, and – (dK) is the decrease in the stock of capital every year due to depreciation of existing capital.

Interrelationship among the equations:

Equations (2) to (4) are closely linked and together describe how the capital stock (K) changes over time. These three equations enable us to calcu­late total saving first, then relate saving to new investment, and, finally, describe how new investment changes the size of the capital stock.

5. The Labour Supply Equation:

We assume that labour force grows exactly as fast as the total population. This is a fairly accurate assumption in the long run.

So the labour supply equation is expressed as:

∆L = n x L … (5)

where n is the growth rate of both population and labour force and ∆L is the change in the labour force.

The model has five equations and five variables (Y, K, L, I and S). So it can be solved. In addition, there are three parameters (d, s and n) the values of which are assumed to be fixed exogenously, or outside the system.

Since the aggregate level of saving (in equation 2) directly determines the level of invest­ment in equation 3, which (together with depreciation) determines changes in the capital stock in equation 4, we get the following equation by combining equations 2, 3, and 4

∆K = sY-dK … (6)

This equation simply states that the change in the capital stock (∆K) is equal to saving (sY) minus depreciation (dK). This expression allows us to calculate the change in the capital stock and enter the new value directly into the aggregate production function.

The Harrod-Domar Growth Model:

The aggregate production function—which is the main pillar of every growth theory—can take different forms, depending on the actual relationship between the factors of production (K and L) and aggregate output. The Harrod- Domar model is based on the simple fixed-coefficient pro­duction function of the Leontief type. In this case, the isoquants are L-shaped, in which case K and L are always used in fixed proportion to produce different levels of out­put, as is shown in Fig. 1.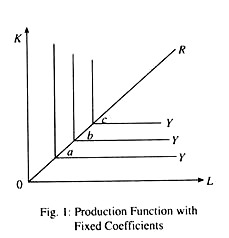

The production function is the ray OR which connects points like a, b, c, i.e., the elbow of each isoquant. With CRS the isoquants will be L-shaped and the production function will be a straight line through their minimum combination points. In this case, both capital-output ratio and labour-output ratio remain constant.

The Capital-Output Ratio:

The Harrod-Domar model was developed during the forties to explain the relationship between growth and unemployment in advanced capitalist societies. The central focus of the model is on the role of capital accumulation in the growth process. This is why the model has been extensively used in LDCs to examine the relationship between growth and capital re­quirements.

In this model, output is assumed to be linear function of capital as:

  Q = 1/v.K or Q = K/v…..(7)

where v is a constant. In eqn. (1) the capital stock is simply multiplied by the fixed number 1/v to calculate aggregate production.

Eqn. (1) can be also be expressed as:

V = K/Q……(8)

so v is the capital-output ratio. It is essentially a measure of the productivity of capital or investment.

Two things get reflected in the capital-output ratio: capital intensity and efficiency.

It is the reciprocal of the average product of K:

 

A high value of v implies more capital- intensive production activities. Therefore, those countries which have a large share of produc­tion in capital-intensive activities (such as steel, machinery, petrochemicals or automobiles) will show a larger aggregate capital-output ratio than a country that specialises in labour- inten­sive industries such as agriculture, textiles, food processing and footwear.

A high value of v can also imply less efficient production because it indicates how effi­ciently a society is able to utilise its present capital stock. In this model, since v is assumed to remain constant, the average capital-output ratio is the same as the incremental capital-output ratio (ICOR). The ICOR measures the productivity of additional capital.

It is often interpreted as the reciprocal of the marginal physical product of K:

The production function eqn. (1) can be converted into another equation to relate changes in output to changes in the capital stock

∆Y = ∆K/v

The growth rate of output, g, is simply the increment in output divided by total output . Dividing both sides of eqn. (3) by Y, we get

g = ∆Y/Y = ∆K/Yv ……(10)

Since the change in the capital stock AK is equal to saving minus the depreciation of capital (∆K = sY-dK) from eqn. (9), get, by substituting eqn. (6) into eqn. (4), following relationship between capital stock and growth

g = s/v ………(11)

This is the basic equation of the Harrod-Domar growth model, from which we can make the following two predictions:

1. The stock of capital crested by an act of investment in plant and equipment is the man determinant of growth.

2. Saving (both by households and companies) makes investment possible. Equation (10) brings into focus two key determinants of the growth rate — the saving rate and the efficiency with which capital is used in production or the productivity of investment (v).

So the central message of the Harrod-Domar model is that if a country saves more to make productive investments, its economy will continue to grow.

Application of the Harrod-Domar Model:

It is very easy for planners and policymakers to apply the Harrod-Domar model. They are left with two alternatives:

Alternative 1:

The first step is to estimate v and d for the country. Then a target rate of growth of the economy (g) can be fixed. Then the equation will tell the economic policymakers the level of saving and investment necessary to achieve that growth.

Alternative 2:

The policymakers can decide on the rate of saving and investment that is feasible or desirable. Then the equation will tell them the rate of growth in national product that can be expected.

The model can be applied to the economy as a whole, or to each sector or each industry. The value of v can be estimated separately for agriculture and industry. Once planners decide how much investment will be allocated to each sector, the model will enable them to determine the growth rates that can be expected in each of the two sectors.

Strengths and Weaknesses of the Harrod-Domar Model:

Over short periods of time (a few years) and in the absence of severe economic shocks (such as drought or large changes in export or import prices), the model can be used to estimate ex­pected growth rates easily and quickly. This is precisely the reason why this model has been extensively used in developing countries for economic planning.

However, the model has several limitations. The most serious is that in this model, the economy remains in equilibrium (with full employment of both labour force and capital stock) only in some special circumstances.

Since the production function is of fixed co efficiency type, capital stock and labour force must always grow at the same rate to main­tain equilibrium. But this is unlikely to happen. In order to keep v constant, K must grow at the rate g—which is the rate of growth of output. If K grew faster or slower than g, v would change.

Let us suppose that the labour force grows at rate n which is exactly the rate of population growth. Therefore, only if n = g = (s/v – d) then the capital stock and labour force will grow at the same rate. However, there is hardly any reason to suppose that the population will grow at the rate n.

On the one hand, if n > g, the labour force is growing faster than the capital stock. In this case, s is not high enough to support investment in new machinery sufficient to absorb all new additions to the labour force. So there will be the problem of unemployment (labour redun­dancy).

On the other hand, if g (or s/v -d)the capital stock is growing faster than the labour force. In this case, there will be shortage of manpower and some machines will remain idle. So actual growth rate will be n, which is less than g. The slowing down of the growth rate is due to non-availability of workers required to operate the machines fully.

In short, unless g = s/v – d, or exactly equal to n, either labour or capital will not be fully employed and the economy will not be in a stable equilibrium. This characteristic of the model is known as the knife-edge instability problem.

In short, as long as g = n, the economy remains in equilibrium. But as soon as either the capital stock or labour force grows faster than the other, the economy falls over the edge with growing unemployment or idle (machine) capacity.

The instability problem arises due to the assumptions of fixed capital-output and capital- labour ratios, which do not permit equalisation of g with n.

This lack of flexibility of the model is its most serious limitation. Moreover, the constancy of v is a reasonable assumption in short run but not in the long run. When the economy evolves and develops v may also rise or fall due to policy changes which affect efficiency with which capital is used.

Moreover, the capital-intensity of the production process may change over time. A low-income country with a low savings rate and surplus labour can achieve faster growth rates by making the maximum possible utilisation of its surplus labour and minimum amount of scarce capital.

With economic growth and rise in per capita income, there is less and less surplus labour in the economy and a gradual shift towards more capital-intensive production. Consequently, the ICOR increases. Thus, a rise in the value of v does not necessarily imply inefficiency or slower growth.

Fixity of ICOR:

Thus, the Harrod-Domar model tends to become more and more inaccurate over extended periods of time as the actual ICOR changes and with it the capital-labour ratio. These changes may occur to changes in wage rate and interest rates in response to changes in market forces (demand and supply conditions of labour and capital).

With economic growth the saving rate rises, and so the rate of interest or the price of financial capital falls while employment and wage rise. As a result, the production process becomes more capital-intensive since all producers increasingly economise on labour and use more capital and the ICOR tends to rise.

Lack of Factor Substitution:

Due to fixed coefficient type of production function, there is no scope for substitution of capital for labour or vice versa in the Harrod-Domar model. More output cannot be pro­duced by hiring one more worker without buying a machine or by purchasing one more machine without hiring some workers.

However, as the neoclassical growth theories, pre­sented by Solow and Meade, have convincingly demonstrated, the knife-edge instability problem can be solved by permitting factor substitution which is possible at least to some extent in the real world.

Technological Change:

Finally, there is no mention of any technological change in Harrod-Domar model. Technologi­cal progress plays a crucial role in the long-term growth and development by raising the pro­ductivity of existing resources. Technological progress can be shown by an inward shift of each isoquant towards the origin. The easiest way to capture technological progress in the Harrod- Domar framework is to introduce a smaller ICOR, but this would contradict the basic assump­tion of the model — constant ICOR.

Joan Robinson: The Accumulation of Capital:

Joan Robinson discussed the importance of capital accumulation to the growth process in 1956, the same year in which Solow’s Work on growth was published.

The Neo-Classical Growth Models:

By combining variable factor proportions and using flexible factors R. M. Solow overcame the Harrod-Domar problem and showed that the growth path of output was not inherently unstable. If the labour force grew faster than the stock of capital, the wage rate would fall relative to the interest rate; while, if capital outgrew labour, the wage rate would rise.

Changes factor prices in directions made intuitively plausible by the presumed operations of market forces could miti­gate the likely deviations from the Harrod-Domar growth path. We may now discuss two neo-classical models which rescued the Harrod-Domar model from its inherent instability problem, viz., the Solow model and the Meade model.

1. The Solow Model:

Neoclassical growth theory refers to general term referring’ to the models for economic growth developed in a neoclassical framework, where the emphasis is placed on the ease of substitu­tion between capital and labour in the production function to ensure steady-state growth, so that the problem of instability found in the Harrod-Domar growth model because of the as­sumed fixed capital to labour coefficients is avoided.

Solow explored the behaviour of the economy as it steadily grows through time. In particular, he looked at the relationship between labour force growth, capital growth and technological growth and examined whether the growth process has any inherent tendencies to slow down.

Solow wrote a paper in 1956 on balanced growth paths along which the growth rate of capital exactly equals the growth rate of labour, so that the amount of capital available for each worker neither rises nor falls.

As Joan Robinson has put it, ‘The rate of technical progress and the rate of increase of the labour force govern the rate of growth of output of an economy that can be permanently maintained at a constant rate of profit.’

In fact, the long-run growth model was introduced for the first time in that because it was built on the classical models used by economists before Keynes. The Solow analysis makes extensive use of the production function and a simple assumption about saving.

Saving and Balanced Growth:

In the simplest version of Solow’s neoclassical growth model, the economy is closed (so do­mestic saving equals investment) and there is no technological change. These two assumptions make it easier to see what is going on in a modern capitalist economy. Labour-force growth is assumed to be at a constant rate, n. Each year the labour force increases by n times N, the level at the start of the year.

The change in the capital stock equals net investment. If capital is to grow at the rate, n, then each year capital must rise by the amount nK. In order to stay on a growth path where the capital stock grows at rate, n, net investment must be nK each year. We can think of nK as balanced growth investment

For example, if the capital stock is Rs 10 million and n is 1 per cent, then net investment must equal 1,00,000 times Rs 10 million if the capital stock is to grow at the same rate as labour.

Here, the first key Condition for balanced growth is:

Net investment = nK . . . (1)

The second major element of Solow’s analysis deals with saving. Saving depends on (i) the fraction of national income that is saved, and (ii) the level of national income. Let s be the fraction of income that is saved, sY is called the saving level. Saving in the economy is equal to s times income. Since income equals output, Y, we get

Saving = sY … (2)

For example, if income Y is Rs 5 million and the saving rate is .02, then saving would be Rs 1, 00,000. Since paving equals net investment, we see that sY equals the actual amount of net investment in the economy.

A subsidiary assumption of Solow’s growth analysis is that the production function has constant returns to scale. Under constant returns and with unchanging technology, if there are equal proportional changes in labour and capital, output changes by the same proportion.

The neoclassical production function is expressed as:

Y = F (K, N, T) … (3)

We could divide K, N and Y by any number and the production function would still apply with constant returns. We choose to divide by N.

This has the effect of stating output as output per worker, Y/N, and capital as capital per worker, K/N:

Y/N = F (K/N, 1, 7) … (4)

Example:

Suppose Y = F (K, N, A) = K^1/3 N^2/3 A. Divide by N to get Y = (K/N)^1/3 -(N/N)^2/3

T=(K/N)^1/3 1.A = F (K/N, 1,A).

In other words, we replace AT with (K/N) and we replace N with 1 in the production function. Output per worker depends just on capital per worker, since we are assuming that technology, T, is constant over time.

Actual investment can be either greater or less than balanced growth investment. Solow developed a famous diagram to explain what happens in the two cases. The diagram is shown in Fig. 2.

It shows the amount the economy saves per worker (the curving line), and the amount of investment per worker needed to keep the capital stock growing at the same rate as the labour force (the straight line). The steady state occurs at the intersection where saving generates just the right amount of investment to stay on the balanced growth path. If capital per worker is less than the steady-state level, investment exceeds the amount needed for balanced growth, and the amount of capital per worker rises. Hence, the economy tends towards its steady state.

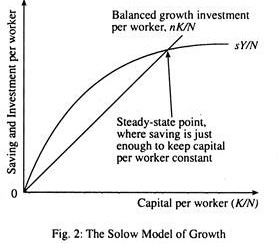
The straight line in Fig. 2 expresses Solow’s conclusion about the amount of net investment needed to keep capital growing at the same rate as labour grows. The total amount of net investment is nK, so the amount per worker is nK/N. Because the horizontal axis is capital per worker, K/N, the amount of net invest­ment—n times YK/N)—is a straight line with slope n.

The curving line expresses Solow’s conclusion about saving per worker. Total sav­ing is sF(K, N, T), so saving per worker is sF (K, N, T )/N which we can also write as sF (K/ N, 1, T). The line is curved because it is a con­stant 0) times the curved production function.

The intersection of the investment line and the saving curve in Fig. 2 is the steady-state point. At this point, the actual amount of investment, determined by saving, is just the amount needed to keep the capital stock growing at the same rate as labour input is growing. If the economy starts at the steady state, it will stay there.

What happens if the economy starts with less capital per worker? This would correspond to a point to the left to the steady-state point in Fig. 2. Saving per worker, and thus actual invest­ment, exceeds the amount needed to keep capital per worker constant. Each year capital per worker increases.

The economy will gradually approach the steady-state point. Similarly, if the economy starts with more capital per worker than the steady-state amount, capital per worker will decline each year and the economy will approach the steady state. Solow showed that the growth process is stable. No matter where the economy starts, it will converge over time to the same steady state, with the capital stock growing at the same rate as the labour force.

The Effect of Saving on Growth:

Another important conclusion from Solow’s work is that, in the longer run, the growth rate does not depend on the saving rate. In the steady state, the capital stock and output both grow at the same rate as the labour force. The only factor that matters for the rate of growth of the economy is the growth of labour input. Economies that save more do not grow faster in the longer run.

What then is the impact of increasing the saving rate in the Solow analysis?

Suppose that the saving rate suddenly rises from .02 to .04 and stays there. Then the bal­anced growth condition is violated with K/Y = 2 s/n = 4. According to Solow’s stability argu­ment, capital will increase more rapidly than labour and because of diminishing returns to capital, the capital-output ratio increases.

The ratio will continue to increase until it reaches 4 and the economy returns to the balanced growth rate of 1 % per annum. There is a transition period, however, during which the growth rate of the economy is greater than the balanced growth rate. Hence, greater saving benefits the economy by raising future GDP, but not by increasing the long-term growth rate, according to the Solow model.

Criticisms:

Much of the criticism of Solow’s and other versions of neo-classical growth theory focuses on its aggregate production function. Influential critics, such as Robinson and Kaldor, have argued that the microeconomic concept of the production function cannot be realistically aggregated to an entire national economy. (Samuelson has shown a link between the microeconomic and macroeconomic production function, but it is not general.) In addition, the flexibility of the neoclassical production function is argued to be unrealistic.

Machinery as capital, for example, cannot be reduced in size as the employment of labour increases. Furthermore, the disembodi­ment of technology from capital is considered to be unrealistic because technological progress is intertwined with capital improvements. Recent work by Romer has extended the neo-classical model so that technology is considered a separate factor of production. Romer considers technol­ogy, or scale.

So increasing returns, as illustrated by the high productivity tendencies of the rich countries, cannot be accommodated easily by conventional neo-classical models in which factor prices are determined in the kind of competitive markets associated with constant returns to scale. Increasing returns are commonly associated with monopolistic markets rather than competitive ones. Unfortunately, the “best” aggregate production function remains to be decided, and both the two-factor version and its extensions provide good empirical fits with reality.

2. The Meade Model:

The neo-classical explanation of economic growth had been extended by James Meade in 1962. His model considers a single aggregated output which can be used either for consump­tion or capital formation. Meade takes the production function in which output is a function of three inputs. So the general form of the production function is

Y = f (K,L, R, t)

where K, L and R are, respectively, capital, labour and land and t stands for time representing a constant trend of technological improvement. Each of the factors of production is easily related to overall output. When any one or any combination of them grows, the output will increase as well.

To be more specific, when land is a fixed factor of production while both labour and capital can grow and time, here taken as a proxy for technological improvement, marches on, changes in output can be expressed in terms of changes in the inputs to the production process:

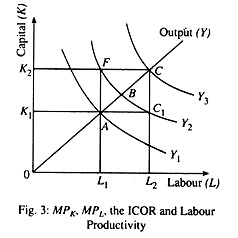
ΔK = vΔK + wΔL + ΔY’ where v is the MP_K, w is MP_L and AY’ the improvement in output attributable to technological change. Here v differs from the reciprocal of ICOR (AK/AY) because it measures the increase in output due to an added unit of capital, holding all other inputs constant. The same ceteris paribus conditions are not present in the application of ICOR. Similar differences separate the marginal productivity of labour (w) and the incremental labour-output ratio. Fig. 3 shows these differences.

The MP_k is represented by the increase in output associated with increasing capital from K₁ to K₂ without changing the quantity of labour L. It is thus the distance AB divided by the distance K₁K₂ (B being on the same isoquant as F). This is less than the reciprocal of ICOR, shown as the distance AC divided by the distance K₁K₂.

The essential difference between the two concepts in the ceteris paribus assumption is made while defining the MP_K. The same statement holds with respect to the MP_L and labour-output ratio.

In Meade’s model, growth in output (which remains an undifferentiated homogeneous quan­tity) can be expressed in terms of the growth rates of the various inputs:

ΔY/Y = ΔK/Y+ ΔK/K+ wL /Y. ΔL/L +ΔY’/Y

where ΔY/Y , ΔK/K, ΔL/L ΔY’/Y are proportionate rates of growth in annual terms of income, capital, labour and technical progress. In fact, vK/ Y is the elasticity of output with respect to capital and wL/Y is the elasticity of output with respect to labour.

Thus economic growth rate is the elasticity of output w.r.t. capital, or its relative contribu­tion to output, times the rate of growth of capital stock; plus the elasticity of output w. r. t. labour, representing its relative contribution to output, times the rate of growth of labour force (or of worker-hours); plus the rate of growth of output owing to technological change.

The most important difference of the neo-classical model for the H-D model lies in its use of changing relative factor prices and productivities to change the factor proportions – i.e., the proportion in which inputs are combined in the production process.

A fall in wage rate leads to substitution of capital by labour which is not possible in the H-D model, because it is a fix-price model. The neo-classical model is based on the implicit assump­tion that the forces of competition within the economy are so strong that employers are sufficiently sensitive to these price changes. So they respond by changing their production techniques.

The Kaldor Model:

The two problems—one of the H-D model, viz., the inherent instability (or the knife-edge problem) and the other of the neo-classical model (the implication of instant and complete adjustments to factor price changes through factor substitution)—were overcome simultane­ously by Nicholas Kaldor in 1957.

He objected to the neo-classical assumption of ready substi­tution between capital and labour due to the rigidity of the technology already embodied in existing machines. For Kaldor, all technological change is embodied in physical capital. No technical progress can occur without accompanying investment.

In contrast, all technical change in the neo-classical model is disembodied in the sense that it proceeds as time marches on— with or without supporting investment. Such a technical progress occurs when an industrial engineer rearranges the existing machines in a new plant layout and thus produces a larger volume of output without increasing the stock of capital.

According to Kaldor, the escape from instability is tied to the relations uniting technical progress and capital-output ratio. If technical progress were to occur much faster than the capital stock, the MP_k would increase, leading to more investment. The converse is also true: if capital investment proceeded faster than technical change, the MP_K would fall, discouraging such a rapid rate of investment.

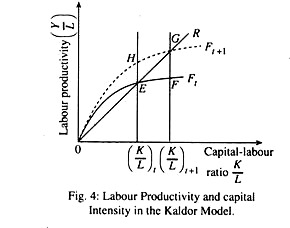
The relationship is illustrated in Fig. 4. The production function F_t shows the possibilities for labour productivity as a function of the capital-labour ratio K/L- in period t. The curve becomes flatter as increasing capital/labour ratios lead to a fall in MP_K (due to diminishing returns to capital). When the slope of F_t is zero, MP_K = 0.

In the next period (t + 1), technical progress lifts the possibilities for labour productivity to F_t+1. At every level of the capital-labour ratio, MP_K has increased. The slope of F_t+1, is steeper at H than the slope of F, at E. Further investment is likely to take place to restore the former MP_k (and the former capital-output ratio) at G.

Let us suppose instead that increased investment between period t and t + 1 moved the capital-labour ratio from E to F along an F, unaffected by technological change. The decline in MP_k would discourage further investment. Only replacement of existing machines as they wore out would be made, until the capital-output ratio was restored by technical progress to its old level as shown by the slope of 0G. (Note that the capital-output ratio is the same along the way through the origin 0R).

Economic growth, proceeding according to this mechanism, tends to work along an equilib­rium path in which the growth rates for all three macro-variables, viz. the capital stock, total output and labour productivity are all equal.

Some Stylized Facts about Growth:

Kaldor (1963) listed a number of stylized facts that he thought typified the process of economic growth:

1. Per capita output grows over time, and its growth rate does not tend to diminish.

2. Physical capital per worker grows over time.

3. The rate of return to capital is nearly constant.

4. The ratio of physical capital to output is nearly constant.

5. The shares of labour and physical capital in national income are nearly constant.

6. The growth rate of output per worker differs substantially across countries.

Simon Kuznets brings out other characteristics of modern economic growth. He notes the rapid rate of structural transformation, which includes shifts from agriculture to industry to services.

This process involves urbanisation, shifts from home-work to employee status and, an increasing role for formal education. He also argues that modern growth involves an increased role for foreign commerce and the technological progress implies reduced reliance on natural resources.

Finally, he discusses the growing importance of government —”the spread of modern economic growth placed greater emphasis on the importance and need for organisation in national sovereign units —.” The sovereign state unit was of critical importance as the formulator of the rules under which economic activity was to be carried on; as a referee; and as provider of infrastructure.

Some stylized facts about growth, i.e., those aspects of economic growth that everyone knows or takes for granted are:

1. Economic growth as a process implies that the capital stock grows more rapidly than the labour force Therefore, the capital-labour ratio increases over time.

2. The increasing amount of capital combined with complimentary labour implies that labour productivity, measured simply as the amount of output in a period divided by the labour inputs in the same period, also rises.

3. The relative shares of labour and capital remain constant in the growth process. However, empirical studies show a rise in the share of labour and a fall in the share of capital in national income.

4. The return to capital is constant, or at least shows no definite trend over time. The evi­dence on this point is mixed.

5. The capital-output ratio is constant or, at least, shows no definite trend over time. Evi­dence, however, shows a fall in capital-output ratio over time due to rising productivity of capital, caused by technological progress. At the same time, capital stock has grown more slowly than national income. In the long run, investment as a proportion of national product has fallen.

The savings ratio and the investment ratio have moved in opposite directions in the industrial countries. This paradox could be resolved by allowing for an open economy. Savings can be channelled abroad. So investment here refers to gross domestic capital formation or domestic investment.

6. The savings ratio (or investment ratio) has remained constant.

While public investment has risen in industrial countries over the past century these have been more than offset by a fall in private consumption as a proportion of national income.

Why Study Growth Models?

Growth models enable us to quantify the most basic elements of the actual growth process by showing the relation of the factor inputs to output and to one another as also to highlight the role of technological progress. Some growth models are applied in practice.

For example, In­dia’s Second Five Year Plan (1956-61) was based on the Mahalanobis model which gives a clear direction for intersectoral allocation of resources. But growth models which are abstrac­tions from reality cannot be used to solve the problems of growth.

Endogenous Growth:

In the mid-1980s, a group of economists led by Paul Romer (1986) became almost totally dissatisfied with exogenously driven explanations of long-run productivity growth. They developed a different class of models in which the key determinants of growth were endogenous to the model. The name ‘endogenous growth’ carries the significance that the long-run growth rate is determined from within the model rather than by some exogenously growing variables like unexplained technological progress.

The simplest version of the endogenous growth model, called the AK model (based on the AK type of production first introduced by von Neumann in 1937) is based on the assumption of a constant saving ratio. This model shows how the elimination of diminishing returns can lead to endogenous growth.

The AK Model:

The main property of endogenous growth models is the absence of diminishing returns to capital. The production function without diminishing returns is expressed as

Y = AK … (i)

where A is a positive constant (like the one in the Cobb Douglas production function), that is, an index of the level of technology. Here K may be treated in a broad sense to include both physical and human capital so as to assume away the absence of diminishing returns to capital in the AK production function. Output per capita is y = Y/L = A. K/L= Ak and the AP_L and MP_K are constant at the level A > 0.

In the Solow model the growth rate of capital is given by

Y_k k/k = sf(k)/k –n-δ ……(ii)

Here we use the symbol y to denote the growth rate of any variable, s is MPS, k = K/L capital per capita, n is the rate of population growth and δ is the rate of depreciation.

If we substitute f(k)/k A is equation (ii), then we get

Y_k = sA = (n + δ) … (iii)

It is now possible to show that per capita growth can now occur in the long run even without exogenous technological change. Now in case of the AK model the downward-sloping curve, sf(k)/k is replaced by the horizontal line at the level sA as shown in Fig .5.

This means that Y_k is the vertical distance between the two lines sA and n + δ. If the technology is AK, then the saving curve sf(k)/k is a horizontal line at the level sA. If sA >n + δ then k grows in perpetuity, i.e., Y_k > 0′ even in the absence of technological progress.

Since the two lines are parallel, Y_k is constant. To be more specific, it has no functional relation to k. Alternatively stated, k always grows at the steady-state rate, = sA – (n + δ).

Since y = Ak, y_y also equals Y*_k at every point in time. Furthermore since per capita con­sumption c = (1 – s) y, where 5 is the saving rate, the growth rate of consumption equals Y_k. This means that all the per capita variables in the model grow at the same rate, given by

Y = Y*= sA-(n + δ) …. (iv)

Thus an economy characterised by the AK technology can display positive long-run per capita growth even in the absence of exogenous technological change. Furthermore, the per capita growth rate in equation (iv) depends on the behavioural parameters of the model, such as the savings rate and the rate of population growth. For example, unlike the neo-classical model, a higher saving rate, 5, leads to a higher rate of long-run per capita growth, Y*.

Alternatively, if the level of technology, A, improves once and for all or if the elimination of a governmental distortion effectively raises A, then the long-run growth rate is higher. Changes in the rate of depreciation, 5 and population growth, n also have permanent effects.

Comparison with Solow Model:

Unlike the Solow model, the AK formulation does not produce absolute or conditional conver­gence, that is dY_y/dy = 0 for all levels of y. This is a major defect of the AK model because conditional convergence is empirically verified almost regularly.

Let us suppose some economies are structurally similar in the sense that the parameters A, n and δ are the same. The economies differ only in terms of their initial capital stocks per person, K (0) and, hence, in Y (0) and C (0). Since the model predicts that each economy grows at the same per capita rate, Y*, regardless of its initial position, all the economies are supposed to grow at the same per capita rate. This conclusion emerges due to the absence of diminishing returns.

Another central idea of the endogenous growth theory is that the level of the technology can be advanced by purposeful activity, such as R & D expenditures.

As R. Barro and X.S.I Martin put it:

“This potential for endogenous technological progress may allow an escape from dimin­ishing returns at the aggregate level, especially if the improvements in technique can be shared in a non-rival manner by all producers. This non-rivalry is plausible for advances in knowl­edge, that is, for new ideas.”