How to Derive Indifference Curves through Revealed Preference Approach?

Revealed preference approach provides us an alternative method to the Hicksian introspective method of deriving indifference curves of a consumer.

In the Hicksian method, indifference curves are obtained by asking the consumer to express his preference among all possible combinations or baskets of two commodities.

However it has been found that consumers are unable to provide reliable answers to direct questions about their preferences between various baskets or combinations of goods.

Further, in the Hicksian analysis some stringent assumptions are made about consumer for deriving indifference curves. For instance, in the Hicksian method of deriving indifference curves it is assumed that consumer is able to give ranking to or order consistently all possible combinations of two commodities. This implies that consumer has a complete knowledge of his precise preferences between various baskets of goods. This is indeed a very strong assumption about a consumer.

On the other hand, in revealed preference approach it is not needed to assume that consumer can rank or order his preferences or to provide other information about his tastes and likings. It is worth noting that in Samuelson’s revealed preference approach, no assumptions about consumer’s preferences or tastes are made.

In fact, in revealed preference approach information about consumer’s preferences is inferred from observing his actual behaviour or choice of goods in the market. Thus, in revealed preference approach we can derive indifference curves of consumers from observing his behaviour in the market or his actual choice of commodities in the market.

For deriving indifference curves by means of revealed preference hypothesis we make the following assumptions:

1. Choices of a consumer are consistent. This implies that if a consumer is once observed to prefer combination A to combination B, then he will never prefer B to A.

2. Consumer’s tastes and preferences remain constant over a time period his behaviour is being observed.

3. Consumer’s preferences are transitive. This means that if a consumer prefers basket A to basket B, basket B to basket C, then he will prefer A to C.

4. A consumer behaves rationally in Pareto sense, that is, he prefers more of a good to a less of it.

In the procedure to derive indifference curves with revealed preference approach we make repeated experiments by varying prices of goods and observe consumer’s choices. It is assumed that consumer’s chosen combination of goods is preferred by him over all other alternative combinations which he could afford to buy. Thus, the combinations of goods not chosen would be either those which are inferior (in terms of ranking or preferences) or those which lie outside the budget space.

Now, consider Figure 12.9 where AB is the initial budget line with a given income and prices of the two goods. Suppose it is observed that the consumer chooses combination R. It can be inferred from this behaviour or choice of the consumer that he prefers R to other baskets of goods lying on the budget line AB or within the budget space. Thus, with the given price-income situation AB, when the consumer chooses R it is inferred that all other combinations of goods which he can afford to buy are revealed to be inferior to his chosen combination R.

Besides, we can infer more from consumer’s observed choice. As it is assumed that a rational consumer prefers more of both the goods to less of them or prefers more of at least one good, the amount of the other good remaining the same, we can infer that all combinations lying in the rectangular shaded area drawn above and to the right of chosen combination R are superior to R since in the rectangular shaded area there lie those combinations (baskets) which contain either more of both the goods or at least more of one good, the amount of the other remaining the same.

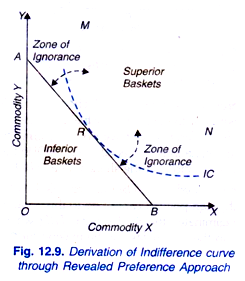
This means that the consumer would prefer all combinations in the rectangular shaded area to the chosen combination R. In other words, all combinations in the shaded area MRN are superior to the chosen combination R and therefore cannot lie on an indifference curve (which represent baskets of goods yielding the same level of satisfaction) passing through the chosen combination R.

As seen above, all other combinations lying in the budget-space OAB in Fig. 12.9 are attainable or affordable but are rejected in favour of R and are therefore revealed to be inferior to R. We now have some information about the location of indifference curve passing through the chosen combination R and other combinations of goods yielding equal satisfaction as combination R.

This indifference curve passing through R will lie somewhere in between the budget line AB and the shaded area MRN drawn directly above and to the right of point R. The region in between the budget line AB and the shaded area MRN is known as zone of ignorance within which an indifference curve can possibly lie.

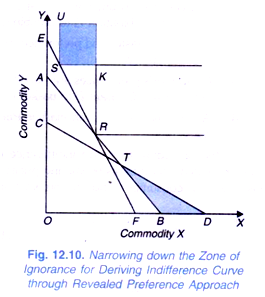
To find the exact location of the indifference curve in the zone of ignorance, we can narrow down this zone of ignorance by making changes in prices of the goods and then observing consumer’s choice. This is illustrated in Figure 12.10 where initially budget line is AB and consumer chooses point R. As explained above, all combinations lying above and to the right of point R will be preferred to the chosen combination.

And all other combinations lying on the budget line AB and to the left of it are shown to be inferior to R since the consumer chooses R when they are affordable. In order to reduce the zone of ignorance let us assume that price of X falls so that the new budget line is CD. The consumer will not choose any combination to the left of T on the budget line CD because such a choice would be inconsistent as combinations on CD to the left of T being below the initial budget line AB are inferior to T.

Therefore, the consumer will choose either T or any point to the right of T on the segment TD. Suppose the consumer actually chooses T on the budget line CD. Using the postulate of transitive preference we have R is preferred to T in the initial budget situation, 7is preferred to the combinations in shaded area (TBD) in the new budget situation. Hence, R is preferred to the combinations in the shaded area (TBD).

It follows from above that combinations in the shaded area TBD are revealed to be inferior to point R and therefore indifference curve through point R cannot go to this area. In this way from the lower zone of ignorance we have eliminated the shaded area (TBD) where the indifference curve through R cannot be located.

In the similar fashion by making further experiments of reducing price of good X and observing consumer’s choice in the new situation we can eliminate more area where the indifference curve through R cannot possibly go till we find in the lower ignorance zone the exact location of the indifference curve through point R.

Likewise, we make experiments for the points (or baskets of goods) lying above and to the left of point R (i.e. in the upper zone of ignorance). For this, to begin with, we draw a new budget line EF which passes through the original chosen point R on the initial budget line AB. On the new budget line EF, the consumer will either choose R or a point such as S to the left of R.

Suppose the consumer actually chooses combination S and thus reveals his preference for S to the combination R. Using Pareto’s rational assumption that a person prefers more to less of a good, all the baskets (combinations) of goods in the shaded area USK would be preferred to the basket S since the baskets of goods lying in this shaded area as compared to S contain either more of both the goods or more of one good, the amount of the other remaining the same. We now have

Baskets in (USK) are preferred to S

Basket S is preferred to the basket R

Hence, by transitivity, baskets in (USK) are preferred to R.

Thus, we have been able to rank combinations in (USK) as preferred to R and therefore indifference curve through R cannot pass through the shaded area (USK). In this way we eliminate the area (USK) from the upper zone of ignorance. We can continue making more such experiments for eliminating more areas and narrowing down the zone of ignorance till we find the exact position of the indifference curve through R. Hence revealed preference hypothesis enables us to derive indifference curve from the actual choice and behaviour of the consumer in different market situations.

Convexity of Indifference Curve and Revealed Preference Approach:

In the Hicksian ordinal utility analysis we prove the convexity of a typical indifference curve on the basis of diminishing marginal rate of substitution. The importance of revealed preference approach also lies in that it enables us to establish the convexity of indifference curve without involving the diminishing marginal rate of substitution.

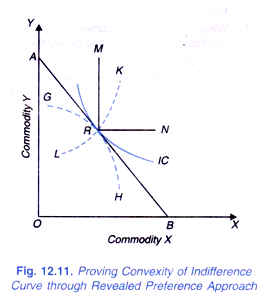
This is shown in Figure 12.11 where we have drawn a budget line AB on which the consumer chooses basket R. As seen above, all baskets of goods on the budget line AB or below it are revealed to be inferior to R and all those baskets in the area (MRN) are revealed to be superior to the basket R. Further,as explained above, the indifference curve through R must lie somewhere in the zone of ignorance.

Now, the indifference curve cannot be straight line AB since all other baskets of goods, lying on the line AB are revealed to be inferior to the basket R when this R is chosen and therefore the consumer cannot be indifferent between R and any of them.

The indifference curve cannot be a curve such as LK cutting the budget line AB at R since this would imply the point on LK below R as being indifferent with R while the consumer has already revealed is preference for R over them when he chose R. Further, points on RK, as explained above, are superior to R and cannot therefore lie on an indifference curve passing through R.

The indifference curve also cannot be concave such as GH through R because all points on it have been ranked inferior to R when choice of R is made by the consumer (Note that all other points on GH contain less of the goods as compared to R).