Williamson’s Model of Managerial Discretion

Williamson argues that managers have discretion in pursuing policies which maxi­mise their own utility rather than attempting the maximisation of profits which maximises the utility of owner-shareholders.

Profit acts as a constraint to this managerial be­haviour, in that the financial market and the shareholders require a minimum profit to be paid out in the form of dividends, otherwise the job security of managers is en­dangered.

The managerial utility function includes such variables as salary, security, power, status, prestige, professional excellence. Of these variables only the first (salary) is measurable. The others are non-pecuniary and if they are to be operational they must be expressed in terms of other variables with which they are connected and which are measurable. This is attained by the concept of expense preference, which is defined as the satisfaction which managers derive from certain types of expenditures.

In par­ticular, staff expenditures on emoluments (slack payments), and funds available for discretionary investment give to managers a positive satisfaction (utility), because these expenditures are a source of security and reflect the power, status, prestige and pro­fessional achievement of managers. Staff increases are to a certain extent equivalent to promotion, since they increase the range of activity and control of managers over resources. Being the head of a large staff is a symbol of power, status and prestige, as well as a measure of professional success, because a progressive and increasing staff implies successful expansion of the particular activity for which a manager is responsible within a firm.

Managers’ prestige, power and status are to a large extent reflected in the amount of emoluments or slack they receive in the form of expense accounts, luxurious offices, company cars, etc. Emoluments are economic rents accruing to the managers; they have zero productivity in that, if removed, they would not cause the managers to leave the firm and seek employment elsewhere.

They are discretionary expenditures which are made possible because of the strategic position that managers have in the running of the business. Emoluments are probably less attractive than salary payments since there are certain restrictions in the way in which they may be spent. However, they may have tax advantages (since they are tax deductible) and furthermore they are less visible remunerations to the managers than salary, and hence are less likely to attract the attentions and cause dissatisfaction of the shareholders or the labour force of the firm.

Finally the status and power of managers is associated with the discretion they have in undertaking investments beyond those required for the normal operation of the firm. These minimum investment requirements are included in the minimum profit con­straint together with the amount of profits required for a satisfactory dividend policy. Discretionary investment expenditure gives satisfaction to the managers because it allows them to materialise their personal favourite projects. This is an obvious measure of self-fulfillment for managers and top executives.

Staff expenditures, emoluments and discretionary investment expenses are measurable in money terms and will be used as proxy-variables to replace the non-operational concepts (power, status, prestige, professional excellence) appearing in the managerial utility function. Thus the utility function of the managers may be written in the form

U = f₁ (S, M, I_D)

where S = staff expenditure, including managerial salaries (administrative and selling expenditure)

M = managerial emoluments

I_D = discretionary investment

Basic Relations and Definitions:

The Demand of the Firm:

It is assumed that the firm has a known downward-sloping demand curve, defined by the function

X = ƒ*(P, S, Ɛ)

or

P = MX, S, Ɛ)

where X = output

P = price

S = staff expenditure

Ɛ = the condition of the environment (a demand-shift parameter reflecting autonomous changes in demand)

It is assumed that the demand is negatively related to price, but positively related to staff expenditure and to the shift factor ε. Thus

An increase in staff expenditure is assumed to cause a shift in the demand curve upwards and thus allow the charging of a higher price. The same holds for any other change in the environment (e, for example an increase in income) which shifts upwards the demand curve of the firm.

The Production Cost:

The total production cost (C) is assumed to be an increasing function of output

Actual profit II:

The actual profit is revenue from sales (R), less the production costs (C), and less the staff expenditure (S)

Π = R – C – S

Reported profit Π_R:

This is the profit reported to the tax authorities. It is the actual profit less the mana­gerial emoluments (M) which are tax deductible

Π_R = Π – M = R – C – S – M

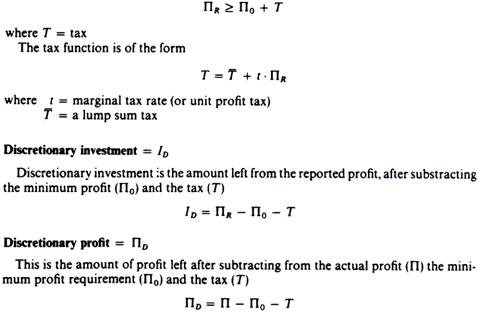
Minimum profit Π ₀:

This is the amount of profits (after tax) which is required for an acceptable dividend policy by the shareholders. If shareholders do not receive some profit they will be inclined to sell their shares or to vote for a change in the top management. Both actions obviously reduce the job security of managers. Hence they will make sure to have a minimum profit Π₀ adequate to keep shareholders satisfied. For this the reported profits must be at least as high as the minimum profit requirement plus the tax that must be paid to the government

 

Implications of the Model:

The implications of this model will become clear if we compare it with the model of a profit maximiser.

There will be no slack payments to managers or discretionary investment if a firm is a profit maximiser, while both M > 0 and I_D > 0 in Williamson’s model.

The expenditure on staff will be larger in Williamson’s model

Sw > S_Πmax

since the profit maximiser will spend on staff up to the point at which an additional unit expenditure on staff will bring to the firm a revenue equal to unity (MC on staff = MR from staff), while the utility-maximising managers will employ staff beyond that point. This follows from the second-equilibrium condition (∂R/∂S < 1 and ∂R/∂S = 1 for Williamson’s firm and the profit maximiser respectively).

Although the first condition for equilibrium is the same in both models (MR = MC), no definite prediction can be drawn about the level of output. In general the optimal X will not be the same in the two models, given that output depends on S, staff expenditures, and these will be larger in Williamson’s model. One might draw the inference (from S_w > S_Πmmx) that X_w > X_Πmax, but this is not necessarily true. The result of increased staff expenditures on the level of output depends on the particular relationship between X and S in the two models.

In summary, staff expenditure, managerial slack and discretionary investment spending will be larger for a firm that maximises utility than for a firm that maximises profits. However, no general conclusion can be drawn regarding the level of output in the two models. (Note: Williamson’s model yields identical results to those of a profit-maximising model if ρ = 1 and the marginal utility for staff (U₁) is zero. Thus Williamson’s model includes the profit-maximising firm as a special case).

Comparative Static Properties:

We may compare further the two models by examining their predictions about the changes of the policy variables (the reaction of firms) when some change in the environ­ment takes place. We will examine the effects on the levels of the policy variables (X, S, ρ) of a shift in demand (change in the shift factor Ɛ), a change in the profit tax rate, t, and of the imposition of a lump-tax T to the firm. We will examine only the direc­tions of these changes.

A Shift in the Market Demand:

This may be denoted by a change in the shift factor ε, appearing in the demand function.

The effects of such a shift on X, S and p in the two models are shown (without proof) below:

A shift in demand will increase output X, and staff expenditure S, in both models. The two models give identical predictions of the direction of changes in X and S, so that by observing only changes in these variables in response to a shift in demand we cannot tell whether the firm is a utility-maximiser or a profit-maximiser. The two models cannot be verified by simply looking at what happens to X and S as demand changes.

However, an upward shift in demand, while not affecting ρ in a classical model, will lead to a reduction in ρ if the firm is utility-maximising. A shift in demand will increase slack payements faster than the increase in the actual profits (Williamson argues). Thus an increase in slack payments in booms, and a decrease of slack in recessions, suggest that the firm is of the Williamson type rather than a profit maximiser.

An increase in the profit tax rate t:

The effects of this change on X, S and p are summarised below:

An increase in the profit tax rate will not change the equilibrium X and S of a profit- maximising firm. A profit-maximising firm cannot avoid the burden of an increase in the profit tax rate by changing its output (or its price) or its staff expenditure, unless the burden is so high as to lead the firm to close down. A utility-maximising firm, on the other hand, will be able to avoid part of the tax burden by increasing its staff expenditure and its slack payments, and reporting a lower level of profit for taxation.

Effects of the imposition of a lump-tax T:

The effects on output, staff expenditure and on slack payments are summarised below:

The imposition of a lump-tax will not change the short-run equilibrium X and S of a profit-maximising firm, which cannot avoid the burden of T (unless it is so heavy as to make it more profitable to close down). On the other hand, the imposition of T leads a utility maximiser to a reduction of his output, reduction of his staff expenditure and reduction of the slack payments. (The decrease in X will occur as a result of a shift downwards of the demand curve, due to the cut in staff expenditure.)

Change in fixed costs:

Since a lump-sum tax is similar in its impact on the firm’s activities to an increase in its fixed costs, we may infer from the above analysis that an increase in the fixed costs will not affect the short-run equilibrium X and S of a profit maximiser (unless he is driven out of business completely), while it will lead to a change in the level of output, the staff expenditure and the slack payments of a utility maximiser.

Empirical Evidence:

Williamson conducted several tests of his model. We will summarise the evidence he presented from several case studies.

Principal-firm analysis:

Williamson attempted to test the hypothesis that managerial discretion influences the expenditures for which managers have a strong expense preference (staff expenditure, emoluments, discretionary investment). He applied ordinary least squares to the model

where X_i = compensation of the top executive of the ith firm

S_i = staff expenditure (administrative, general and selling expense)

C_i = concentration ratio in the industry where the ith firm belongs

H_i = height of the barriers to entry in the industry

B_i — composition of the board of the ith firm (proportional representation of the management in the board)

U_i = random variable

He fitted the above model to cross-section samples for the years 1953, 1957 and 1961. Each sample included the two largest (principal) firms (ranked according to sales) from twenty-six industries. The samples were not random, but included the large firms for which the managerial model is thought to be more appropriate.

Surprisingly enough the dependent variable is only a small fraction of staff expendi­ture, not any one or all of the expenses for which managers have a definite preference. Williamson argues that the remuneration of the top executive is determined within a carefully designed scale for the salaries of the rest of the managerial group. ‘Payments between executive levels are carefully scaled so that the factors which influence com­pensation of the top executive can be presumed to affect the level of staff compensation generally.’ (Williamson, ‘Managerial Discretion and Business Behaviour’).

Even more questionable is the use of S, staff expenditure, as a determinant of the re­muneration of the top executive. Williamson uses S as a proxy for the ‘compensation which the top executive would receive strictly on a profit-maximising basis’, on the grounds that staff expenditure reflects the size of personnel over which the top executive has responsibility.

This may be so, but the logic for including the profit-maximising remuneration as a determinant of the actual compensation of the top executive does not seem clear to us, given the hypothesis being tested. If anything, staff expenditure, S, is probably the most important element in the discretionary expenses of managers, and, hence, should be the dependent rather than an explanatory variable.

The concentration ratio, C_i, and the height of the barriers to entry, H_i, are used as measures of opportunities for managerial discretion. Williamson argues that the higher the concentration (the fewer the firms in an industry) and the stronger the barriers to entry, the greater the power of managers for discretionary spending. Williamson, anticipating the criticism that C_i, and H_i, are another measure of ‘size’, estimated the correlation coefficient between sales and C_i, and sales and height of barriers to entry.

He found the values of these correlations sufficiently low (-013 and —014) for the firms included in his samples, and he thus concluded that C_i and H_i are not proxies for size. However, he found that the correlation between sales and S (‘staff expense’) was con­siderable (0-75). Yet, he does not comment on the implications of this correlation for his estimated regression.

Another criticism might be the simultaneous use of C_i and H_i as measures of the opportunities of discretion for managers. Why is neither of these measures adequate for capturing the effect of ‘opportunity for managerial discretion’? Williamson does not answer this question; hence one might suspect that the combined use of C_i and H_i was chosen in order to improve the statistical fit, rather than on grounds of theoretical importance of these variables in explaining the dependent variable.

Williamson, anticipating the criticism that C_i and H_i are a measure of profitability, estimated several equations in which profits were included either in combination with C_i and H_i or replacing them. From his statistical findings he concluded that profits give a worse fit to his data. We should think that the evaluation of the regression findings on the basis of statistical results alone is not adequate for concluding that C_i and H_i are not in fact a proxy for profits.

The variable B_i, proportionate representation of management on the board, is used by Williamson as a measure of the ‘desire of managers to act free from outside inter­ference’, that is, free from the interference of owner-shareholders. The greater the number of managers on the board, the greater the ‘desire’ of management for discretionary action.

It seems to us that the distinction between the ‘desire of managers’ and the ‘opportunity of managers’ for discretionary behaviour cannot be disentangled and measured independently by the three variables C_i, H_i, and B_i. Obviously one can argue that the greater the representation of management on the board, the greater (not only their desire but also) their opportunity for discretionary action. Thus, we should think that C_i H_i and B_i are largely overlapping measures of the same factor the degree of discretion that managers have in the allocation of resources in the firm.

In summary, the causality implied by staff, S, plus the fact that S and sales are highly correlated, that H_i and C_i may reflect profitability, that C_i H_i and B_i may measure (at least partly) the same factor, cast serious doubt on the suitability of the fitted model for testing the hypothesis of managerial discretion.

Evidence from field studies:

Williamson conducted several case studies from which he infers that his model is better suited for the explanation of some real-world phenomena, such as:

1. Increase in S and M in booms and drastic cut of these expenditures in recessions.

2. Reaction of firms to taxation changes.

3. Changes of the level of X, S and M in response to changes in the fixed costs of the firm. O. Williamson’s Model of Managerial Discretion

4. Drastic cuts in staff expenditure by newly appointed top management, without affecting the productivity of the firm.

5. Allocation of ‘fixed overheads’ of multiplant-multiactivity corporations to their different plants and activities so as to obtain the effects of a lump-tax (that is reduction of inefficient plants and activities).

Such phenomena, Williamson argues, while incompatible with a profit-maximising behaviour, can be explained by his model of ‘rational managerial behaviour’.

We think that the available evidence is not enough for the verification of the theory. The above arguments of Williamson rest on an implicit ‘ceteris paribus’ clause, which is not at all sure to be fulfilled in dynamic situations, such as shifts in demand and costs in booms and recessions.

Furthermore, Williamson’s model fails to deal with the core problem of oligopolistic interdependence and of strong oligopolistic rivalry. Williamson’s model is applicable in markets where rivalry is not strong (for example, in an oligopolistic market where there is some form of collusion), or for firms who have some advantage over their rivals (for example, patents, superior know-how). However, in the long run such advantages which shelter a firm from competition are usually weakened, and competition is en­hanced. When rivalry is strong a profit-maximising model may be more appropriate, unless some form of collusive agreement is achieved and firms adhere to it.