Game Theory: Assumptions and Structure of Game Theory

Game theory was introduced by a mathematician, John Von Neumann and an economist, Oskar Morgenstern, in 1950s.

This theory aims at providing a systematic approach to business decision making of organizations. It is applied to evaluate the situations where individuals and organizations have contradictory objectives.

For example, while settling a war between two nations, every nation tries to get the settlement in its favor only during peace meetings/negotiations.

In such a case, game theory helps in solving the problem and arriving at a common consensus. Apart from this, the theory can be applied to analyze activities, such as legal and political strategies and economic behavior.

Over a passage of time, the game theory has emerged as a vast and complex subject. The games in the game theory are simple as well as complex. The main aim of applying the game theory is to find out the best strategy to resolve a particular problem.

Moreover, the game theory helps organization by increasing the probability of earning maximum profit and reducing the probability of losses. The game theory has applications in sociology, psychology, and mathematics.

Assumptions of Game Theory:

The game theory provides an appropriate solution of a problem if its conditions are properly satisfied. These conditions are often termed as the assumptions of the game theory.

Some of these assumptions are as follows:

i. Assumes that a player can adopt multiple strategies for solving a problem

ii. Assumes that there is an availability of pre-defined outcomes

iii. Assumes that the overall outcome for all players would be zero at the end of the game

iv. Assumes that all players in the game are aware of the game rules as well as outcomes of other players

v. Assumes that players take a rational decision to increase their profit

Among the aforementioned assumptions, the last two assumptions make the application of the game theory confined in real world.

Structure of a Game:

Game theory is based on the concept of strategy and payoffs. Strategy indicates an action that a player takes when challenged to solve a particular problem. On the other hand, payoff refers to the outcome of the strategy applied by the player. For example, two friends are playing coin flipping game.

In this game, one friend tosses the coin, and the other friend calls for head or tail. In case, the caller’s projection about the coin is correct, then he/she gets the coin. However, in case the caller’s projection is wrong, then he/she would lose the coin and the other person gets the coin.

Therefore, in this game, the caller’s projection of head or tail would be regarded as the strategy and the payoff would be the result of coin flipping, which means that either caller wins the coin or tosser wins the coin. In coin flipping game, the outcome or payoff depends on the caller as he/she projected the side of coin. However, in other games, the payoff may depend on more than one player.

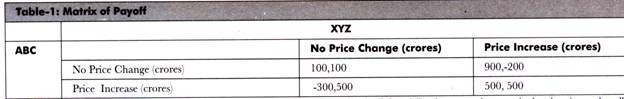
Let us understand the tabular representation of payoff and strategies adopted in a game with the help of an example. Suppose two competing organizations, ABC and XYZ, decide to increase their profits by making changes in the prices of products. In this case, it is assumed that both the organizations can adopt two strategies. One is to increase the price level of their product and another is to maintain the same price level.

As per these strategies, there can be four possible combinations of strategies, which are as follows:

i. Both ABC and XYZ has increased the prices of their products

ii. Only ABC has increased the price of its product, while XYZ has not made any changes in the price level of its products

iii. Only XYZ has increased its prices, while ABC has maintained the constant price level

iv. Both ABC and XYZ have maintained the same price level of their products

Table-1 shows the tabular representation of payoffs and strategies of organizations ABC and XYZ:

In Table-1, the first numerical value of every cell represents the payoff of ABC, while the second numerical value in each cell represents the payoff of XYZ. The tabular representation of strategies and payoffs is termed as payoff matrix. Therefore, in the present case. Table-1 is a payoff matrix for organizations, ABC and XYZ.