Complete Guide to Keynesian Economics

Complete Guide to Keynesian Economics!

Keynesian Economics and the Economics of Keynes:

A prior knowledge of the fundamental ideas of J.M. Keynes is absolutely essential for an understanding of Keynesian economics.

The basic and fundamental ideas on which Keynes’ theory of employment has been built are—effective demand, consumption function, investment, saving, marginal efficiency of capital, multiplier, liquidity preference and under-employment equilibrium.

These related ideas are all brought together into one theory of employment’, which is the essence of “General Theory”. Keynes was basically a classical economist in the sense that his ideas were essentially based on classical readings and teachings. He remained wedded to classical economics for a long lime having taught it for more than twenty years.

His ideas and philosophy can, therefore, be fully understood only when read against the background of classical economics. It was not until Keynes had lived through the great depression that he was able to divorce some of the standard classical doctrines. Keynes wrote, “The classical theory is misleading and disastrous if we attempt to apply to the facts of experience.”

The subject matter of this book, therefore, includes not only the Economics of ‘Keynes’ but also ‘Keynesian Economics’ and important developments after Keynes. In recent years the scientific, as well as the not so—scientific, literature in Economics has been filled with research papers, articles, books—refining, modifying, enlarging, criticizing, supporting and at times refuting—what is now commonly called ‘New Economics’, ‘Keynesian Economics’, ‘Modern Macro theory’, ‘Keynesian Revolution’. The positive contributions made by other writers (who followed Keynes) in this vast literature are equally important.

Thus, on the one hand, an effort has been made in this work to expose in a simple and thorough manner the ideas of the one man (Keynes) who stands out above all others as the chief architect of ‘New Economics’; on the other hand, important refinements and extensions of Keynes’ work have been introduced.

An attempt has been made to survey the supplementary literature on Keynes’ writings and to interpret the numerous debates which have centred round Keynes. Keynes’ Economics of macroeconomic relations have had undergone many changes in the course of discussions that followed—’Keynesians’ or ‘Neo-Keynesians’ depart from original train of thought at a number of points and aim at studying these changes that have taken place. However, difference of opinion exists amongst Keynesians themselves.

Some have stayed closer to Keynes’, original theory than others. Consequently, a difference between orthodox Keynesians and Neo-Keynesians will occasionally emerge in the chapters that follow. Still, there are quite a few who like to stay closer to the anti-classical orthodoxy than others.

In ‘Keynesian’ Economics we study models structurally similar to and based on Keynes’ conceptual framework. Economics of Keynes-primarily his ‘General Theory”, is the foundation on which Keynesian economics has been constructed. Following the publication of his book, economists went through it line by line, accepting, correcting and rejecting. What they have built on the foundation that remained is a massive structure known as Keynesian economics.

In Keynes’ economics, we study the static or comparative static model contained in the ‘General Theory’—but Keynesian economics takes into account the rule of expectations, dynamic and period analysis, importance of various types of lags (time lags, consumption, administrative and expenditure lags) arid thus tries to improve upon the tools and the basic conceptual framework as adopted and developed by Keynes; for example, then is a difference between Keynes’ consumption function and Keynesian consumption function; ‘Keynes’ logical multiplier and Keynesian dynamic multiplier. Similarly, there is a difference in Keynes’ approach to saving and investment, income and expenditure, liquidity preference, rate of interest, demand for and supply of money and ‘Keynesian’ approach to these concepts. Thus, the newer ideas developed after Keynes as a result of other influences, and the models built there on, are designated as ‘Keynesianism’.

It may be true, that some economists may resent being called ‘Keynesian’ ; even then, almost all would agree that there does exist today a recognizable majority view on the theory of income determination and that the term can reasonably be used to refer to the main outlines of this view. Keynesian economics, in this popular sense, is far from being a homogeneous doctrine.

However, the main contention is that Keynes’ theory is quite distinct from ‘Keynesian’ theory—as it is possible to describe (at least in rough outline), a model (or class of models); with a substantially different structure from that of income-expenditure model, that is more consistent with the textual evidence of Keynes’ major work. The income-expenditure model has long provided the basic framework for the exposition of Keynes’ system and for comparing Keynesian with Classical theory as well as the General theory with later versions of Keynesian doctrine.

While the numerous and varied interpretations of Keynes in the last forty years are not identical, the great majority are cast in this mold. Within this broad framework there is, admittedly, ample room for differences to emphasis in the various interpretations of Keynes—but the basic mold is there. Hence, the necessity of defining, understanding and comparing the contents of ‘Keynesian Economics’ to that of the ‘Economics of Keynesian’. His theory may not make us Keynesians, it does make us practical economists.

New Keynesian Concepts:

In recent years there has been a greater use of certain terms specially with the development of post- Keynesian ideas on economic growth. These are sometimes described as tools of macroeconomics analysis.

Such tools or terms are described below:

Variables (Endogenous and Exogenous, Stock and Flow Variables):

A variable is a quantity that may vary over a range. In other words, it is a quantity subject to continual increase or decrease: a quantity which may have an infinite number of values in the same expression. Thus, a variable is easily defined as a measurable or scalable magnitude which varies, and in whose variations we have an interest, partly because of its direct importance and partly because of its effect on other variables. It is interesting to note that these variables vary a lot as a result of which a static system becomes dynamic. Though there are a large number and types of variables— continuous, dependent, independent, detrimental, discreet, random, etc. yet macroeconomics is not interested in studying them all.

However, important amongst them are as follows:

Sometimes, these variables belong to a system as its integral parts when they are called endogenous variables and sometimes they may be external to the system, when they are described as exogenous. Variables explained within the structure of a model or systems are called endogenous variables.

These are the economic variables whose determination is the purpose of the model, e.g. if the model provides how national income is determined, then national income is an endogenous variable. Variables which are not explained, but are taken as given outside the model or from outside the system, are called exogenous variables. Exogenous variables may be either non-economic or economic in nature and are determined independently by the system or model.

These variables are included in the model to show how changes in them influence the system but are not themselves determined within the system. For example, if the money stock is assumed to be determined by the monetary authority and its value given to the system implied by the model, then the money stock is an exogenous variable.

There is no hard and fast line of demarcation between them. Usually, it is a matter of convention as to what variable we regard as endogenous and what exogenous. Population and the changes in its size and composition, imports, techniques of production etc., are generally regarded as exogenous variables, though the possibility of their being endogenous at a different time and under different circumstances cannot be ruled out. For example, population may increase in a fashion that cannot be attributed to the changes in the variables of the system itself, but it is also true that it tends to grow in response to favourable circumstances of the economic system.

Whether a variable is endogenous or exogenous it is either stuck or flow. It is useful to distinguish between ‘Stock and Flow’ variables. A stock variable has no time dimension: whereas a flow variable has. The weight of a bus is a stock variable and its speed a flow variable. The magnitude (amount of the stock) has no time dimension, but a How can only be expressed per unit of time.

The speed of the bus is 80 kms per hour, is a How concept; while there is a fleet of 100 buses with Haryana Roadways, Ambala plying between Delhi and Chandigarh, is a stock concept. The main distinction between the stock and flow variables depends on the time dimensions to which the two relate. A stock variables refers to a quantity measured at any given point (or moment or instant) in time; a flow variable is a quantity which can be measured only over any given period of time. Stock variables have a time reference associated with them; while How variables have a time dimension.

Although both stock and How variables are dated, a stock variable is different from a How variable because a point in time is different from a period of time. The balance-sheet or Stock Statement shows the assets and liabilities of a business firm at a point in time. The profit and loss statement or flow statement shows the receipts and expenditures incurred over a period of time.

The macro How concepts include the national income, output, consumption, investment, saving, wages, interest, profit etc., while the macro-stock concepts include total money supply, total bank deposits, wealth, inventories, capital stock, debts etc. There are certain macroeconomic variables which have both the How and stock magnitudes. For example, money supply is a stock while the changes in money supply are a flow magnitude. Certain other variables like wages, dividends, social security and tax payments and the like are only flow quantities and have no stock counterpart. Similarly, money is a stock and the expenditure flowing from it is flow concepts; income is a flow, wealth a stock.

Again, saving is a flow, while savings is a stock. Investment is a flow, while the aggregate of investment is a stock. However, price is neither a stock alone, nor a flow alone. It is belter to describe it “as a ratio between two flows—a flow of cash and a flow of goods”. Other ratio variables may express relationship between stocks, relationship between flows, or relationships of stocks to flows.

Examples are—ratio of liquid assets to total assets (liquidity), ratio of saving to income, ratio between a flow of money transactions and stock of money (velocity) respectively. In the simplest formulation of Keynesian theory the flow of consumer spending is determined by the flow of income, and the flow of income equals the flow of consumer spending plus the flow of investment spending (K = C + I). However, in more advanced analysis changes in such critical stocks as the stock of money and the stock of capital also affect the flows of income and product.

Functional Relationships and Parameters:

A functional relationship exists between two variables when they are related in such a way that the value of one depends uniquely upon the value of the other. Relationships between variables are described by the term function, consumption is a function of income. Consumption in this case is a dependent variable, whereas income is an independent variable. To say that something is a function of something else does not specify anything more than a relationship.

Consumption could rise or fall with income; it would be a function of income in either case. The responsiveness of one variable to changes in another is an important feature of macroeconomic models. Major arguments in macroeconomic theory involve such issues as the responsiveness of the demand for money to changes in the interest rates of consumption to changes in income and prices and of investment expenditure to changes in marginal efficiency of capital and interest rates.

Economists have started making greater use of the language and apparatus of mathematics. As such, economists now talk of functional relationships amongst their variables instead of vague terms like ‘tendencies’ or ’causes’. The existence of a functional relationship among two or more variables simply means that the value or the magnitudes of the variables are somehow uniquely related. A variation in one variable is bound to affect in a certain fashion the other variable.

We, thus, simplify the analysis by assuming a functional relationship between two variables only. This relationship may be between price and quantity demanded. The ‘Marshallian Demand Curve’ deals with a single relation between two variables which proved extremely valuable in the analysis of price challenges. Given the Marshallian Demand Schedule (function), there is some unique quantity which the buyers will purchase corresponding lo each price.

Hence, quantity demanded is a function of price and may be expressed symbolically as D = f (P); where D is the quantity demanded of the commodity, P its price and the notation ‘f’ simply means the ‘function of’. Similarly, the Keynesian analysis is based on functional relationships. The Aggregate Demand Function relates any given level of employment to the expected proceeds from that volume of employment. The expected proceeds depends upon the expenditure on consumption and on investment. The former necessitates a study of the consumption function and the latter of the investment demand function (Y = C + I).

A constant variable (quantity) is called Parameter. In static analysis, certain parameters such as taxes, income etc. are assumed as given. When we talk of the demand function based on two simple variables (price and quantity), we hold the values of other variable as constant (called parameters) by using the phrase ‘other things remaining the same’ (ceteris paribus).

In other words, the ordinary demand curve shifts when one of these other variables changes, that is if any of the other; ‘factors’ change, we can say that the parameters of the function have also changed. An important parameter of the ordinary demand curve is ‘consumer’s taste’, if this changes, the demand curve will shift upwards.

At the same price more will be demanded than before. Therefore, we can say that when any parameter of the function changes, the curve will also shift. Although the functional concept is an important tool of economic analysis, there are limitations inherent in the effective use of the concept. Firstly, the fact that two variables are related to one another in a functional sense does not mean that the one is the cause of the other. Cause is not easily determined. Secondly, a particular variable may be a function of a number of other variables.

For example, lo say that the quantity demanded of a commodity is a function of its price—does not mean that the quantity demanded cannot at the same time be a function of other variables, besides price. In the case of the law of demand, we might say that the quantity demanded of a good is a function not only of price, but of buyer’s income, price of other goods, available substitutes, future expectations of prices and perhaps a host of other factors.

Thus, the functional relationship may not simply be restricted to two variables but may involve a number of variables, such as:

D = f (P, P₁, P₂, P₃….P_n, K, Y)

The above equation shows that the demand for a particular good (D) is a function of the price of that commodity (P), prices of other goods (P₁, P₂, P₃…P_n, etc.) the tastes of consumers (K) and the income of consumers (Y). The demand for the particular good depends on all these variables and as these independent variables change, D also changes.

The main techniques used to show or establish functional relationships are: Schedules-Graphs- Equations. Schedules are empirical or hypothetical data whose functional inter-connection we wish to establish or investigate. The depiction of functional relationships through schedules is no doubt simple enough, but economists usually prefer to represent these relationships by graphs or equations. Schedules only show the relationships between specific quantities and prices or specific data of any kind. Graphs and equations, on the other hand, show the generalized relationship; the relationship that will cover all quantities and prices or all values of any two things we are interested in.

Everyone is familiar with graphs of one kind or another. Equations are very simple and convenient method of expressing functional relationships, because they allow us to consider the impact of more than one factor at a time.

A typical equation for income consumption relationship may look like this:

C = ƒ (Y. P, M, r)

The equation shows that consumption is a function of income (Y), price level (P), quantity of money (M) and the rate of interest (r). Usually while studying economic or functional relationships, we make the assumption ‘ceteris paribus’ or ‘other things remaining the same’.

In the above equation if P. M and r are assumed to be constant (and as such these are the parameters) then, C and Y are the dependent and independent variables and the equation will be:

C = ƒ(Y, P. M, r) or C = ƒ(Y)

In the above equation P, M and r are indicated with bars above them showing that these variables are constant (parameters), the C is a function of Y, where C is a dependent variable and Y is independent variable. Similarly, the functional relationship between output (O) and employment (N) where other variables like techniques (T) and stock of capital (K) and entrepreneurial behaviour (Q) are kept constant is shown by the equation: O = ƒ (N, T, K, Q ). Here, T, K, Q are constant (parameters), therefore, short-run output function or relationship between output and employment is shown by the equation O = ƒ(N), which means that in the short-run, output level is a function of labour input or employment (N).

Tautologies:

Tautologies are statements that resemble identities but are not quite the something. They are statements that cannot be proved false because there is no empirical or operational way to examine them. Tautologies play an important part in economic thought. There are many tautological statements in Keynes’ ‘General Theory’, for example, Keynes’ ‘Multiplier’ has been described merely as tautological.

Thus, the information conveyed by a tautology may be real and even useful, but it cannot be objectively accepted or rejected in the way that functional relationships can be. Nor can tautologies be accepted as definitions, the way identities can be. They occupy a special position, giving us statements about reality that reflect our beliefs or feelings, but not statements that can be subjected to empirical scrutiny.

Identities Versus Theories (Behaviour):

Identity implies a simple, mathematical and abstract truism. In fact, all that the identity means is the equality between amounts purchased and sold. The identity between saving and investment is precisely of the same nature. For example, personal saving is equal to the difference between personal disposable income and personal expenditure. This is an accounting relationship which follows by the definition of saving and tells nothing about the behaviour of savers. Identity equations being a needless repetition explain nothing.

To say that the amount of rice purchased in New York market on 1st April, 1981 was equal to the amount of the rice sold, explains nothing about rice prices. Thus, identity equations like ‘MV=PT’ and ‘I = S’ hardly tell anything fruitful. These imply that identical relationships amongst different variables hold irrespective of the magnitudes of the variables.

These identities or accounting relationships are merely, tautological, i.e., they do not explain why and how the different variables have assumed the specific values. Sometimes, identities and behavioural equations are written in the same manner with an equal sign (=).

Technically, identities should be written with an identity sign (=). Unfortunately, for generations of students, these are also read as equals.

Since it is important to know the difference between definitions, which d not need proof, and hypotheses, which always need proof, we have to carefully differentiate between the equal sign (=) and the identity sign (=). Whenever we see an equal sign we know that a behavioural relationship is being hypothesized. When we see the identity sign, we will know that a definition is being offered, not a statement about behaviour.

Therefore, behaviour equations must be sharply distinguished from identity equations. A behaviour equation essentially runs in terms of functional relation between variables. These are statements about behaviours—embodying actual rather complicated hypotheses. The familiar demand function is a schedule, relating amount demanded to price.

In other words, this schedule is a statement about market behaviour. To say that demand is equal to supply, is to say nothing. But if one says that demand and supply in the schedule sense are equal, one is definitely saying something purposeful, namely, that if these schedules involve ranges such that they intersect, then the price and quantity (bought and sold) are mutually determined.

Such points of intersections become observable (actual) points as against the other points which are called virtual points. Virtual points represent the normal or desired relationship of variables, it is therefore, clear that the basic distinction between ‘identities’ and ‘equations’ lies in the fact that identities hold what cover be the magnitudes of the variables and need not be, infact cannot be put to empirical investigations or tests. The behaviour equations, on the other hand, are statements or propositions about the economic behaviour which can be tested by empirical investigations.

Lags:

Modern production is based on process which occupies time. For example, if there is an increase in income, it is not necessary that consumption will go up simultaneously; it may take some time for consumption to increase—thus, the gap or the time involved between the increase in consumption and the increase in income is called ‘lag’ or ‘time lag’ in macroeconomics.

These lags include consumption- expenditure lags, wage-price lags, production lags, administrative lags etc. These lags play a significant role in the theory of income, output and employment. The importance of ‘period analysis’ lies in the study of the working of lags. In Keynes theory, the lagging adjustment that the economic system makes in responses lo the introduction of a disturbance is often skipped over.

In other words, Keynes ignored the working and influence of lags and he broadly took into consideration the instantaneous behaviour of macroeconomic variables. However, post-Keynesians took account of the influence of lags on his theoretical tools like consumption and investment functions, liquidity preference, multiplier etc. For example, Prof. Robertson’s analysis involves expenditure lag—where the consumption of today (C_t) is a function of yesterday’s income (Y_t-I).

In Keynes’ basic income expenditure-equation Y_t = C_I + I_t all the variables belong to current time period and no time lag is involved. But according to Prof. Robertson there is an expenditure lag between income and consumption, i.e., consumption of today (C_t) depends upon income of yesterday (Y _t-I), i.e. C_t = ƒ(Y _t-I).

Thus, Keynes equation (Y_t – C_t + I_t), if expressed in Robertsonian terminology becomes:

Y_t = C (Y _t-I) + I_t

The above equation shows an income-expenditure lag. The study of such lags is the essence of the period analysis. If we ignore such lags, it may not be possible to analyse and investigate the process of dynamic adjustments among different economic variables. There are mainly two types of lags involved in economic analysis—expenditure lag and production lag.

The expenditure lag means the lime interval between the receipt of income and its actual disposal as expenditure. If a person gets a lottery today, his income thereby stands increased. A small part of it may be spent today but the rest of its spending may extend over the future time periods. Production lag refers to the time interval intervening between the injection of investment spending and the maturing of a particular project or the receipt of income by entrepreneurs.

When new investment is made time is spend on planning, construction, transportation, marketing of the product and the receipt of income by the producer. Keynes says, “Time usually elapses, however and sometimes much time between the incurring of costs by the producer and the purchase of the output by the ultimate consumer’. The importance of lags is there not merely because these point to the necessity of adjustments between the variables related to different time periods but also because they consider the inter-relationship of the various variables against a very realistic background.

Time-Series and Cross Section Analysis:

There are mainly two ways of analyzing the statistical data—time-series and cross section. Time series—is a set of ordered observations of a particular economic variable, such as price, production, investment, and consumption, taken at different points in time. Most economic series consist of monthly, quarterly or annual observations. Monthly and quarterly series are used in short-term business forecasting.

The data representing values of aggregate consumption and income over many different years are an example of time series data. Data representing values of consumption and income for many different individuals or groups during a given year are an example of cross section data. Cross section data have been used to analyze secular consumption function. Cross section data are obtained from different sections of society like consumers, workers, producers, firms, industries or other geographical regions.

Ex Ante and Ex Post:

Latin phrases meaning ‘beforehand’ and ‘afterward’. In business cycle theory ex ante refers to quantities of investment, savings or consumption defined in terms of action planned at the beginning of the period in question. Ex ante, thus means anything planned or intended, for example, ex ante saving is the amount that people intend to save out of their incomes in a particular period. Ex post refers to quantities of investment, savings or consumption defined in terms of measurement made at the end of the period in question.

Thus, it refers to realized saving, investment or consumption, e.g., ex post saving is the amount that people do actually save in that period. The ex post results may not necessarily be those that are planned at the beginning of the period and the divergence between the two affects the future plans. The concepts of ex ante and ex post were originated by Gunnar Myrdal in his discussion of monetary theory.

Accelerator:

It is widely accepted that the demand for capital goods is a ‘derived demand’ i.e. derived from the demand for consumer goods. The accelerator shows that the demand for capital goods change as a result of changes in the demand for consumption goods and explains how a changes in the output of consumption goods causes an expansion in the production of capital goods used in making these consumption goods. It denotes a theory that a change in the demand for goods induces a change in the amount of machinery needed to produce those goods.

This theory was introduced by J.M. Clark in 1907 to explain proportionately larger variations in investment over the causes of a business cycle than had occurred in the output of consumer goods. Interest in accelerator as a theoretical tool increased after 1936, when it was discovered that it could be combined with Keynesian consumption to formulate self generating models of the business cycle. Thus, acceleration principle can be used as an explanation to show how changes in consumer expenditure may bring about changes in new capital formation.

International Liquidity:

This refers lo current financial assets which have wide acceptability for the settlements of international indebtedness and include reserves like gold, foreign exchange like dollar or sterling; I.M.F. Drawing Rights ; borrowing schemes of the ‘Group of Ten’ like stand-by agreements; Special Drawing Rights (SDRs) and the non-official Eurodollars. The amount of international liquidity is an important factor in the maintenance of stability in exchange rates.

Equilibrium:

Equilibrium or its absence, disequilibrium are concepts familiar to all students of economics. Equilibrium is usually defined in economics as a position of rest, or as a stale of balance between opposing forces, or as a state requiring no change over time. As such, in equilibrium, there are no forces at work which would change, the values assigned to the variables in the problem. Every time period is an exact duplicate of the previous time period. Disequilibrium, on the other hand, means the absence of a state or balance—a slate in which opposite forces produce imbalance. It is a state requiring change over time. Each successive time period differs from the earlier period.

Since in economics we are always dealing with variables whose values change over time, equilibrium is a state of no change over time. It, however, does not mean that economic equilibrium is a state of absolute rest, a motionless state in which no activity takes place; rather, it is a state in which there is a activity or action of a repetitive nature. The state of equilibrium is maintained, even though the forces acting on the system are in a continuous slate of change, as long as the net effect of these changing forces is such as not to disturb the established position of equilibrium.

Just as disequilibrium exists in the market for a single commodity whenever supply is not equal to demand; so also disequilibrium exists in macroeconomic models whenever aggregate output is not equal to aggregate demand. In the market for a commodity, the price of the commodity adjusts itself upward or downward to bring the quantity supplied and the quantity demanded into equilibrium.

In Keynesian macroeconomic theory, it is the level of aggregate output or income that adjusts upward or downward to bring aggregate output and aggregate demand into equilibrium. Just as the changes in the supply of and demand for a commodity produce a new equilibrium price (or under certain conditions a state of continuous disequilibrium) similarly, changes in consumption and investment propensities produce a new equilibrium output (or under certain conditions a state of continuous disequilibrium).

The concept of equilibrium is a valuable tool of macroeconomic theory because it identifies a position in which the values of the model’s variables are in balance. This helps to simplify the complexity of the real world, where these same variables may actually be in continuous short and long-run disequilibrium. Disequilibrium is also a valuable tool of theory but in a different sense.

Intact, it may be said that short-run equilibrium analysis is a maximum in simplification and long-run disequilibrium analysis is a minimum in simplification. Although we are essentially concerned with equilibrium analysis yet the more difficult branch of macroeconomic theory treats the systems in long- run disequilibrium by admitting into the analysis continuing changes in both flows and stocks.

Markets and Transactors:

Macroeconomics is quasi-general equilibrium analysis. It is concerned with the interrelationships existing among variables whose values are determined in the relatively small and therefore, manageable number of markets obtained after aggregating of the component supplies and demands. In the chapters that follow we shall work with a model consisting of commodity market, labour market, and the bond market and the money market.

The market for commodities is characterized by a supply function, a demand function, and a price of commodities. In terms of macroeconomic variables, the total supply of commodities is equal to real income Y; and the aggregate demand for commodities is equal to the sum of consumption demand, investment demand, and the government demand for commodities (C + I + G).

The price of commodities is equivalent to P. The labour market is characterized by a demand function, a supply function and a price of labour (wage). In terms of macroeconomic variables the supply of labour is equal to total labour force (F), the demand for labour is equal to the level of employment (AO, and the price of labour is equal to real wage (W/P).

The market for commodities and labour together comprise what is described as the ‘real sector’ of the economy. But our economy or model also contains two financial assets—bond and money. The bond is a financial asset whose price varies and money is a fixed price asset. The market for these two assets is called ‘monetary sector’ of the economy. The bond market is also characterized by a demand function, a supply function and the price of the bonds.

Money is a good which has no market of its own. The main feature of money is that it is a good which is exchanged against all other goods in their markets. For example, if a transactor goes to the commodity market as a demander, he offers to buy commodities in exchange for money; and if he goes there as a supplier, he offers to sell commodities in exchange for money. There is always certain supply of money in existence which must be held by transactors collectively.

When the supply of money that must be held is equal to the demand for money to hold (as an asset and for transactions purposes), then we say (though broadly) that the money market is in equilibrium. The price of a unit of money itself is always fixed at unity, since it always takes just one rupee to buy one rupee. If there are four goods in a model (commodities, labour, bonds and money), then there are three independent prices—so many rupees per unit of commodity, so many rupees per unit of labour and so many rupees per unit of bonds but there is always just one rupee per unit of money, i.e., per rupee.

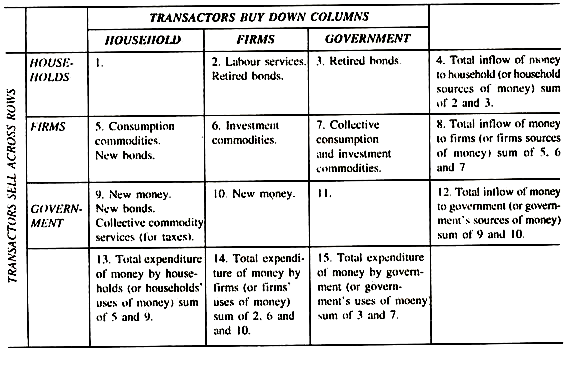
We assume that there are three classes of transactors: households, firms and the government. The transactions in which these groups engage may be summarized in the transactions matrix or flow of funds statement shown on the next page. It shows the market relationships which the three groups of transactors have with each other. Moving across a row one sees what one transactor sells to the other transactors; moving down a column one sees what one transactor buys from other transactors. It is useful to go through this matrix cell by cell.

Cell 1 indicates that households do not sell anything to other households. Cell 2 shows that households sell labour services to firms, and they also sell bonds to firms when those firms choose to retire them. Cell 3 reveals that households sell bonds back to the government when the government chooses to retire them.

Cell 4 is the sum of cells 2 + 3. It indicates the total inflow of money to households. All the money which flows into households must come from the sale of labour services to firms or the sale of bonds bank to firms or the government as either of these transactors retire some of their bonds. Cell 5 shows that firms sell commodities for consumption purposes to households, and they also sell newly issued bonds to households.

Cell 6 indicates that firms sell investment commodities to other firms to be added to the capital stock. Cell 7 reveals that firms also sell commodities to the government. These commodities are either collectively consumed or added to the community capital stock. Cell 8 indicates that the total inflow of money to firms comes from the sale of commodities to the three potential purchasers—households, firms and government and the sale of newly issued bonds to households. Cell 9 shows that governments make available to households the services of the public- good commodities without direct charge. Government is assumed not to sell these services, since it is not able to identify or catch the individual beneficiaries.

But the government needs money to finance its public-good purchase programme. It obtains these funds by collecting taxes, selling new bonds, and issuing new money. Cell 10 has only entry, “New money”. The government may finance the part of its commodity purchase programme by paying firms with some of its new money issues. Cell 11 is empty, since the government engages in no transactions with itself. Cell 12 indicates that the total inflow of money to the government is comprised of the sum of items in cells 9 and 10.

Cells 13, 14 and 15 are the sums of the respective columns above them. Just as moving down a column indicates the purchases that the relevant transactor is making, these sums show the transactor’s total money expenditure. For example, households spend money on consumption commodities, on new bond acquisitions, on taxes and on additions to their money holdings. Broadly similar observations hold for the purchase of firms and the government.

Transactions Matrix or Flow of Funds Diagram:

Economic Progress—Economic Development and Growth:

Economic Development:

Although the terms economic development, economic growth and economic progress are used interchangeably, yet sometimes fine distinctions are made between them. Economic development means economic growth. More specifically it is used to describe not the quantitative measures of a growing economy but the economic, social or other changes that produce the growth. It requires changes in techniques of production, in social attitudes and institutions.

Economic Growth:

Economic growth means economic development. More specifically it may he used to describe the outward evidence of the process of economic development. Growth is measurable and objective e.g., it describes expansion in the labour force, in capital, in the volume of trade and in consumption; whereas economic development is used to describe the underlying determinants of economic growth, such as changes in attitudes and institutions. Economic growth is long-term concept.

It is the rate of expansion of the national income or total volume of production of goods and services of a country. The study of the business cycle is concerned with the change in the extent to which a given production capacity is utilized. In the long-run the expansion of capacity must also be taken into consideration. Economic growth could, therefore, be defined as a constant raising of production capacity.

In other words, it is an increase in a nation’s capacity to produce goods and services coupled with an increase in production of these goods and services. Usually, economic growth is measured by the annual rate of increase in a nation’s gross national product, as adjusted for price changes. A better measure, however, is the increase in the real gross national product per capita; for in some underdeveloped countries yearly gains in output are surpassed by gains in population, leaving the average person with a lowered standard of living.

Economic Progress:

On the other hand, the meaning of economic progress implies value judgments on what is desirable or undesirable say in the distribution of income. It is a wide term which includes in its scope economic development plus also something more—say the value judgments—on what is desirable or undesirable e.g. an economy may be facing economic development but little economic progress, if there is an increase in national income but this national income is unequally distributed or is concentrated in a few hands. Value judgments imply individual decisions not based on rational calculations or logic.

Economic Model:

Economic model is a set of equations or relationship used to summarise the working of the national economy or of a business firm or some other economic unit. Models range from the very simple to the very complex; they may be used to illustrate a theoretical principle to forecast economic behaviour. Some econometric models used in the study of national economic activity comprise several hundred equations.

Economic models are usually theoretical constructions the bulk of economic theory consists of models which, if well devised, identify the influences to be taken into account in the real world and the kind of results to be expected from changes in then According to Gardner Ackley. “An economic model consists simply of a group or set of economic relationships, each one of which involves, at least one variable also appears in at least one other relationship which as a part of the model—Economic models, then, are succinct statements of economic theories.” According to Macdougall, “an economic model is a simplification of reality.” It deliberately submerges detail so as to highlight those processes and relationships that are of greatest importance for the problem at hand.

Macroeconomists frequently attempt to describe the complicated behaviour of the economy by means of a few simple relationships that show how the major aggregate variables are related to each other. These models may consist of mathematical equations, they may be a set of diagrams, or they may take the form of a scheme such as the flow chart of the computer programmer or the electronics engineer.

The devising of simplified skeletal system generally called ‘model’ has become a significant part of the writings on economic theory in recent years. It is the most efficient means of organising an argument, inconsistencies and logical errors are most easily detected. Economists have resorted to model building because they are unable to conduct controlled experiments. They must, therefore, isolate from real situations the variable influences and relationships which are believed to be main determinants of particular results. Having chosen the parts, interconnections and prime movers of a model, they analyse the way in which it works and the changes which additional or different parts and interconnections would make.

If a model reproduces important features of real life it provides a guide to understanding and a basis for predictions. The role of mathematics in building aggregate economic models is that of providing a language for the development of successive degrees of approximations of the real world within which we can examine the impact of various policy alternatives. The ‘Marshallian Demand Curve’ is a simple model which deals with a single relation between two variables.

‘The Marshallian Cross’ consisting of two relationships, a demand curve and a supply curve, is another valuable model. After the appearance of the General Theory, construction of models helped in explaining and understanding Keynesian ideas. Beach remarks, “Had Keynes himself specifically constructed a model in mathematical terms, his ideas might have been appreciated more quickly.” These mathematical economic models became more popular with the works of J.B. Clark, Fisher, Walras, Edge-worth, Chamberlin, Mrs. Robinson, Harrod and Kaleeki to mention a few. These economic models provide an opportunity for prediction, for carving out paths of economic development.

Models may incorporate individual economic units such as households and firms, often grouped into individual markets and industries and the relationship between them. These are called microeconomic models. They help to explain such matters as the determination of prices and outputs of particular commodities and payments for individual factors of production. Macroeconomic models have been extensively developed from the construction of total or national income accounts by the late Sir Arthur Bowley, by Collin Clark and late Lord Stamp, and from the theoretical work of Keynes and the Swedish economists.

These ignore details, and build up systems of broad aggregates, such- as total consumption, total investment, national income and changes in the general level of prices. Macroeconomic models are used in an effort to explain and predict the performance of the economy as a whole, e.g., changes in the level of national income, the level of employment and inflation. The British Treasury uses a macroeconomic model in constructing the budget to estimate its likely effects on the performance of the economy.

Planning bodies use macroeconomic models when working out the implications of alternative rates of growth. Macroeconomic models provide a device by which the aggregate behaviour of the economy is analysed in terms of employment, interest rates, income, prices, balance of payments etc. A model is not a complete representation of reality, it is an abstraction of only those elements which are essential to predict the behaviour of those economic variables the model builders want to study. A macro model typically describes the aggregate behaviour of consumers, investors and holders of money. The simplest model consists of a single equation; in fact, the art of model building lies in getting the maximum amount of information out of the simplest possible model. W. Leontief has devised input-output models which have both microeconomics and macroeconomics features and show the sources of the inputs of different industries and the destination of their output.

They are intended to help the detailed investigation of the effects of general changes, such as reduction in total government expenditure following disarmament. They have so far been constructed only for groups of industries, and for inputs and outputs related by constant proportions.

Both microeconomic and macroeconomic models may be sub-divided into ‘equilibrium’ and ‘process’ models. Equilibrium models specify the conditions under which the ‘variables’ they incorporate would have no tendency to change; but they are used to analyse change. First, they sometimes indicate the direction which adjustment will take in disequilibrium conditions. Secondly, comparison of equilibrium with initial conditions differing in a single respect may indicate the ultimate effect on the single difference.

An important distinction in the case of microeconomic models is between general and partial equilibrium models. General equilibrium models embrace all the variables of the whole economy; their main purpose is to provide a summary chart of the interrelationship between all parts of the economic system.Partial equilibrium models select a few closely interrelated ‘variables’ (e.g.. the price of a single commodity and quantity of it demanded) and workout the mutual interdependence of these few against a background of assumed fixed values for all other ‘variables’.

Equilibrium models ignore the difficulty of tracing the way in which movement is made towards the equilibrium position. But the equilibrium itself may be affected by the path followed and the speed with which different variables change. ‘Process’ model trace out such paths of adjustment indicating the conditions under which steady movement, oscillation and rebounds from ‘ceilings’ and ‘floors’ are to be expected. A further distinction may be made between models which incorporate expectations as determinants of behaviour from those which do not. Non-expectational models work mechanically, expectational models allow for the fact that economic activity takes place in a world of imperfect knowledge.