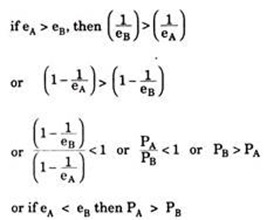The following points highlight the two main conditions necessary to make price discrimination profitable. The conditions are: 1. Elasticities of Demand Must be Different 2. Marginal Revenues must be the Same.
Condition # 1. Elasticities of Demand Must be Different:
Elasticity of demand for the monopoly product in different markets must be different. Market which is insensitive to price changes or relatively less elastic (but not inelastic) must have a price greater than the market in which demand is relatively more elastic.
If elasticities of demand for the monopoly product in two markets are same (i.e., iso-elastic) then uniform price would prevail. Thus, for profitability of a discriminating monopolist, what is required is the difference in elasticities of demand.
Condition # 2. Marginal Revenues must be the Same:
Price discrimination will be profitable only when marginal revenues in different markets are the same. It is true that as these two markets have different demand curves- implying different values of elasticities of demand—marginal revenues may not be the same.
ADVERTISEMENTS:
However, the monopolist can increase his total revenue by transferring his product from the market that has lower marginal revenue to the market that has higher marginal revenue. When the process of transfer of a commodity from one market to another market brings about an equality in marginal revenues of the two markets, price discrimination becomes profitable.
Let us assume that our monopolist sells his product in two markets—A and B.
The above-noted conditions for profitability can be expressed in the following notational form:
a.eA ≠ eB, and
ADVERTISEMENTS:
b. MRA = MRB
where eA and eB are the elasticities of demand for the monopoly product in markets A and B, and MRA and MRB are the marginal revenues in markets A and B.
Now, a discriminating monopolist has to take two decisions in order to attain maximum profit:
(i) How much should he produce, and
ADVERTISEMENTS:
(ii) How should the output be allocated in two markets, and what price he should charge.
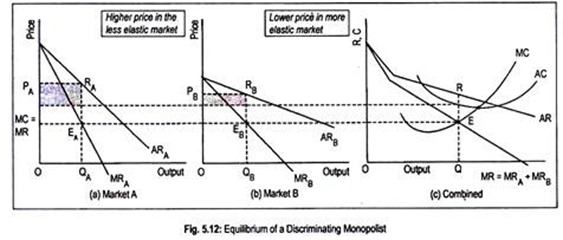
Like an ordinary monopolist, a discriminating monopolist reaches equilibrium when MC = MR. Here, equilibrium condition should be MC = MRA, MC = MRB, and MC = MRA + MRB. In panel (a) of Fig. 5.12, we have drawn the demand curve and marginal revenue curves of market A as ARA and MRA. Panel (b) shows the same for market B as ARA and MRB.
By combining these ARA and ARB curves we obtain ‘combined AR curve’, represented by AR shown in panel (c) of Fig. 5.12. Corresponding ‘combined MR curve’ has been represented by MR = MRA + MRB in panel (c). For equilibrium of the discriminating monopolist, MR must be equal to MC.
This occurs at point E in panel (c). In the aggregate market, the monopolist produces OQ output. If there were no price discrimination, the monopolist would have charged a uniform price, QR, for all buyers. But, price discrimination is more profitable.
Now the task of the monopolist is to allocate the total product OQ in markets A and B in such a way that marginal revenues in all markets are the same (i.e., EQ = EAQA = EBQB). So, OQA must be sold in market A and OQB in market B (since OQ = OQA + OQB). Now the monopolist will charge price OPA in market A for the output OQA and OPB in market B for the output OQB.
Total revenue that the monopolist will earn from the sale of OQA in market A is OPARAQA and from market B total revenue is OPBRBQB. If these two revenues are added together (i.e., OPARAQA + OPBRBQB), it would exceed the total revenue of the monopolist (OQ x RQ). The shaded portions in panels (a) and (b) describe the profit enjoyed by the discriminating monopolist in markets A and B.
Thus, price discrimination is profitable. Further, it is to be remembered here that in the market in which elasticity is comparatively low, price would be higher, and the market in which elasticity is comparatively high the price would be lower. As market A is less sensitive to price changes compared to market B, price in the former market is higher than the latter (i.e., OPA> OPB).
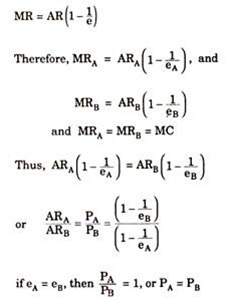
The relationship between elasticity of demand and the policy of price discrimination can be established with the help of the following formula. We know that
This means that if Elasticities of demand are uniform in all markets then it will not be possible and profitable to discriminate between buyers. The monopolist, in this case, will charge the same price to all buyers.
On the other hand,
Thus the monopolist will charge a higher (lower) price in the market in which demand is less (more) elastic.
ADVERTISEMENTS:
However, the coefficient of Elasticities of demand for the product in all the markets must have a value greater than 1 since the monopolist always operates at the elastic range (e > 1) of his demand curve.
If elasticity of demand in market A is 0.9 (i.e., inelastic), but in market B it is 1.1 (i.e., elastic), price discrimination will be unprofitable since MR in market A will be negative and, in market B, positive. Equilibrium solution does not exist.