Investment Multiplier: Concept, Process and Limitations

Investment Multiplier: Concept, Process and Limitations!

Change in Equilibrium National Income: (Static) Investment Multiplier:

The time has come to pose a question of direct importance to the policymakers since aggregate demand is inadequate to put the economy on to the path of full employment. Fig. 10.11 or 10.12 tells us that the economy remains below the state of full employment— the situation of underemployment equilibrium.

So, what is needed is the stimulation of aggregate demand so that new aggregate demand equals aggregate supply or output.

So the question is: What happens to the level of income if equilibrium is disturbed by a change in different elements of injections (i.e., private investment expenditure, government expenditure, or tax schedule)? To ascertain the impact on equilibrium income following a change in injection is called the multiplier.

The multiplier concept is central to Keynes’ theory because it tells us that an increase in investment by a certain amount leads to an increase in income greater than the increase in investment. Thus, an investment has “multiplier effect” on aggregate demand. The concept of multiplier is a solution to the problem of underemploy­ment equilibrium.

While developing his theory of “invest­ment multiplier”, Keynes borrowed the concept from R. F. Khan’s “employment multiplier.” A change in autonomous investment expenditure brings about a change in income. However, the change in income is greater than—or a multiple of— the change in investment.

Suppose, an investment of Rs. 2,000 crore causes an increase in income by Rs. 6,000 crore, then the value of the multiplier would be 3. Thus, the multiplier is the change in income consequent upon a change in investment. Or the multiplier is the ratio of change in income (∆ Y) to a planned change in investment (∆I).

Let the investment multiplier be denoted by K_I. Multiplier is the number by which the change in investment has to be multiplied to obtain the resulting change in income.

Thus,

∆Y = K_I. ∆I

Or, K_I = ∆Y/∆I

Why does income rise in a multiplied form following a rise in investment? From the circular flow of income, we know that business firms earn when households spend, and households earn when firms spend on hiring input services rendered. Thus, total income equals total expenditure. However, a part of this total income is spent on consumption and the rest is saved.

This induced consumption of one individual becomes the income of another individual which, again, results in an increase in consumption.

This, again, creates income—and the process goes on. Thus, an initial autonomous investment expenditure leads to an increase in income via consumption expenditure. However, the process of income generation must stop when the last consumption spending fails to generate fresh income.

Anyway, at the end, the total increase in income will be more than the initial volume of investment. However, how much income will rise in response to an increase in investment depends on the value of MPC or its complementary term, MPS.

The Multiplier Process:

To under­stand the multiplier process, consider the following example. Suppose that the MPC of the community is 0.75 (the value of MPC being greater than zero but less than one) and investment in an industry increases by Rs. 20 crore. This invested amount causes income of the people engaged in that industry to rise also, by Rs. 20 crore.

Since MPC = 0.75, workers of this industry will spend on buying goods produced by the second industry to the extent of Rs. 15 crore (= 20 × 0.75). This consumption of Rs. 15 crore constitutes an increase in income of workers of the second industry.

Consequently, consumption of the people engaged in the second industry would rise. Now consumption would increase by Rs. 11.25 crore (= 15 × 0.75). This constitutes an increase in income of the workers of the third industry.

In this way, the increase in consumption, hence income, triggers still another increase in consumption. This is known as the multiplier process that goes on indefinitely. But since the increase in consumption becomes progressively smaller, the total increase in income becomes finite. The final increase in income following an initial increase in investment of Rs. 20 crore with an MPC = 0,75 is

∆I = Rs. 20 crore

∆ Y= (20 + 15 + 11.25 + 8.44 + ….)

= Rs. 80 crore

Thus, the value of the multiplier is (80/20 =) 4. If MPC = 1/2, an investment of Rs. 20 crore would cause income to rise by Rs. 40 crore. Thus, the value of multiplier would be 2.

The formula for investment multiplier is:

∆Y = 1/1 – MPC. ∆I

Or, ∆Y = 1/MPS. ∆I

If we use the above example, we obtain

∆Y = 1/1 – ¾. 20 = Rs. 80 crore

Thus, greater the value of MPC or lower the value of MPS, greater will be the value of the multiplier. If MPC = 1, the value of the multiplier would be infinite. If MPC = 0, the value of multiplier would be unity. Since 0 < MPC < 1, the value of the multiplier lies between one and infinity.

The logic of the multiplier can also be explained in the way shown in Table 10.2. Suppose there occurs an increase in investment by ∆I. This investment raises income to the recipients of that investment spending. The increased income or output results in an increase in national income.

The increased national income, via MPC (b), gives rise to an increase in aggregate demand b∆I in the second round. Let us assume that output expands by b∆l to meet this increase in spending. Consequently, national output rises. Now this increased national income gives rise to an increase in aggregate demand in the third round by the amount b (b∆ I) = b² A I.

Note that b<1, b²<b. This means that aggregate expenditure in the third round is smaller than the second round. Since the recipients of extra income spend a part (fraction b) and save another part (fraction 1 – b), the process goes on ad infinitum. This has been shown in a table to trace the overall expansion of income following an increase in autonomous investment.

Graphical Exposition:

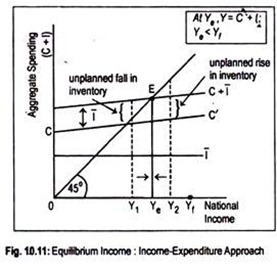
Graphically, the multiplier process, which has a similarity with the ‘ripple effect’ of a stone thrown in a pond, can be illustrated here. In a closed economy without government, equilibrium national income is determined when either (i) C + I line cuts the 45° line, or (ii) saving equals investment.

In Fig. 10.14, CC’ is the initial consumption function and the relevant investment function is I₁. Plotting the consumption and investment functions together we obtain aggregate demand schedule represented by C + I₁. Since this aggregate demand schedule lies above CC’ line at every level of income, it has the same slope as the consumption function.

The vertical distance between CC’ and C + I₁ represents the volume of autonomous investment. The equilibrium level of income, given by the intersection of the 45° line and the C + I₁ line at point E₁, is OY₁. Now, suppose private investment rises from to I₂.

Consequently, aggregate demand schedule rises to C + I₂ and equilibrium occurs at point E₂. Equilibrium national income rises to OY₂. Thus, an increase in investment by AI results in an increase in equilibrium income of ∆ Y. Note that an increase in income (∆ Y) is larger than the increase in investment (∆ I).

The reason behind this process is straightforward. If firms decide to invest more, aggregate demand would now exceed aggregate supply or output. Consequently, inventories would tend to decline. Inventories are part of the capital stock. So, reduction in inventories would act as signal to firms to produce more. Obviously, output or national income would increase to the new equilibrium level.

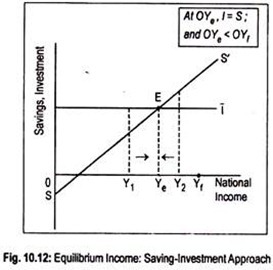
Fig. 10.15 illustrates the graphical exposition of the multiplier process in an alternative way. To be in equilibrium, leakage (here, saving only) must equal injection (here, investment only). Further, investment is assumed to be autonomous, represented by the line I₁ – SS’—the saving schedule—cuts the l₁ line at E₁.

Corresponding to this equilibrium point, equilibrium level of income, thus, determined is OY₁. An increase in investment by an amount ∆I causes the investment line to shift to I₂. Equilibrium point shifts to E₂ and income rises from OY₁ to OY₂. Anyway, the increase in income (∆Y) is bigger than the increase in investment (∆I). The multiplier is now in action and the economy recovers from the ‘Great Depression’.

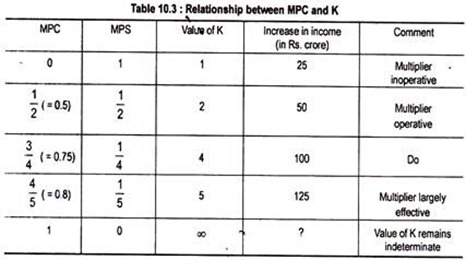
How much national income would increase in response to an increase in investment depends on the value of MPC or its reciprocal, the MPS. Greater the value of MPC or lower the value of MPS, greater will be the value of the multiplier.

Such relationship between MPC or MPS and the multiplier has been shown in Table 10.3. It may be added here that the above result holds only if the consumption function is a linear one (See. Mathematical Appendix: Note 1).

Limitations of Investment Multiplier:

The above discussion suggests that greater the change in investment, greater will be the change in income. However, the Keynesian multiplier analysis is viewed as an ‘ideal’ one in the sense that there occurs an instantaneous adjustment between change in investment and change in income. That is why Keynes’ multiplier is called ‘instantaneous’ multiplier or static multiplier or timeless multiplier.

This multiplier analysis is based on certain assumptions. Firstly, consumption is strictly a function of income and the MPC of the society remains unchanged. Secondly, investment spending is autonomous. Thirdly, the economy remains below the stage of full employment. Fourthly, there is no time lag between income and consumption.

However, in reality, the multiplier process becomes weaker due to the following reasons:

Firstly, Keynes assumed that consumption depends on income and MPC of the economy does not change. But experience and evidence suggest that consumption depends on other factors including income. Keynes ignored other determinants of consumption function.

Above all, MPC does not remain static. Changes in income following a change in investment bring about a change in income distribution which causes MPC to change. MPC for the poor is high compared to the rich people.

In such a situation, it becomes difficult to calculate the value of the multiplier. It is true that 0 < MPC < 1. Suppose MPC is greater than one. If, so then 1/1 – MPC will be negative. This suggests that an increase in autonomous investment results in a decline in national income.

Secondly, the multiplier analysis describes the effect of an increase in autonomous investment on national income. But it neglects the effect of consumption on investment. Changes in consumption result in a change in investment spending.

This sort of investment is called induced investment. Multiplier analysis neglects this aspect. If induced investment is taken into account the value of multiplier will be larger than the simple multiplier presented by Keynes.

Thirdly, multiplier analysis comes to a halt if the economy remains at the full employment level since output or income cannot increase beyond this level even if investment spending increases. Only at the underemployment situation does multiplier work.

Fourthly, Keynesian multiplier is an instantaneous multiplier in the sense that as soon as investment takes place income tends to rise. This is also called ‘static multiplier’ as there is no lag between income and investment expenditure.

However, in reality, there exists a time lag between incomes received and consumption spending. Greater the time lag, lower will be the value of the multiplier because now change in income is not instantaneous. Once we introduce time lag in the process of income change, we get ‘dynamic multiplier’ as opposed to static multiplier.

Finally, leakages or withdrawals result in a smaller value of multiplier. In other words, due to the existence of leakages, the process of income generation slows down. For instance, if people decide to save more from their incomes the value of the multiplier will be weaker.

This is because, in an interdependent economy, more consumption of an individual will result in an increase in income of another individual. Thus, greater the consumption of the community greater will be the value of income.

That is why it is said that investment results in an increase in income via consumption spending. But if society decides to save more (i.e., high MPS) people’s income will decline. In other words, the multiplier process will be weaker if society’s MPS is high.

Again, once we include the government in our analysis the multiplier process may not work in the above-mentioned way. For instance, if the government raises the tax rate or if the corporate sector does not distribute a portion of profit to the shareholders, disposable income will decline. This will cause consumption spending to rise at a slow speed.

Ultimately, increase in income consequent upon an increase in investment will be less. Similarly, if people buy more imported goods, country’s consumption spending for domestically produced goods would be less. Now the resulting increase in income following an increase in investment would be smaller. Thus, greater the leakages (i.e., S + T + M), lower is the value of the multiplier.

Despite these limitations, the multiplier analysis has some uses. Firstly, it demonstrates that a change in investment spending results in an increase in income and employment level. Indeed, it helped the world economy to recover from the Great Depression of the 1930s when an increase in investment spending caused in increase in aggregate output between 1935 and 1940 via the multiplier effect.

However, since private investment alone was incapable of raising the needed output, the recovery was triggered by government spending.

Secondly, by estimating multipliers (for government expenditure, taxes, money supply), it is possible to estimate the effectiveness of fiscal policy and monetary policy. Thirdly, the multiplier concept enables us to analyse cyclical fluctuations, its control and its forecasting. That is why it is said that this concept is a path-breaking one.