Determining Equilibrium National Income (With Example)

The following points highlight the top two methods of determining equilibrium national income. The methods are: 1. Aggregate Income-Expenditure Approach 2. Savings-Investment Approach.

Method # 1. Aggregate Income-Expenditure Approach:

In a two-sector Keynesian model, aggregate demand is composed of planned or desired consumption demand and planned investment demand. The total of planned expenditure (C + I) must be equal to the value of output or income for a simple economy to be in equilibrium.

Or when the C + I line cuts the 45° line, an equilibrium level of income is determined. In other words, an equilibrium level of national income is determined at that point where aggregate demand (C + I) equals aggregate supply (i.e., the country’s aggregate output or national income).

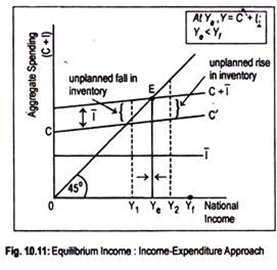
To illustrate equilibrium national output graphically, we use Fig. 10.11 where we measure national income on the horizontal axis and aggregate demand or spending (C + I) on the vertical axis. The 45° line is purely a reference line; any point on this line is equidistant from both the horizontal and vertical axes.

Aggregate spending (C + I) is equal to the value of income or output on this 45° line. For our exposition purposes, this line can be thought of as an aggregate supply curve, though it is not a ‘true’ aggregate supply curve.

With no government and foreign trade sectors, aggregate demand/expenditure is the sum of consumption demand and investment demand. In the Fig. 10.11, CC’ is the planned consumption line. It shows the level of consumption for each level of income. Investment expenditure is assumed to be autonomous.

To demonstrate this, investment line I̅ has been drawn parallel to the horizontal axis. By summing up the consumption and autonomous investment schedules one obtains aggregate demand schedule (C + I̅). The vertical distance between the CC’ line and the C + I̅ line measures the volume of autonomous investment.

Point E is the equilibrium point, since C + I̅ line cuts the 45° line at that point. Equilibrium level of income, thus determined, is OY_e since it is the only level of income at which aggregate demand and aggregate value of output (or income) are equal to each other.

That is,

Y = C + I̅

We can show that this equilibrium level of income is a stable one. This means that if the level of income is either more than or less than OY_e, then there will be a tendency for the level of income to move toward OY_e. Otherwise, equilibrium is said to be unstable. Suppose, if income is OY₁ (< OY_e), aggregate demand will exceed aggregate supply or aggregate output.

That is, C + I̅ > Y

Now there will be an excess demand for goods and services, resulting in an unplanned reduction of inventories. (Remember that inventories are part of investment.) As inventories decline, business firms step up production. Thus, output will continue to rise till OY_e is achieved. Similarly, at OY₂ level of income, aggregate supply exceeds aggregate demand.

That is, Y > C + I̅

This means an excess supply of goods and services. People are not willing to purchase all the goods that the nation has produced. Thus, there will be an unintended accu­mulation of inventories by producers. Firms will now be forced to cut back production. Output will continue to decline till OY_e is attained. Only at OY_e there is neither unplanned accumulation nor depletion of inventories. Thus, OY_e is in stable equilibrium.

To have a stable equilibrium of income one condition is needed—slope of the line (C + I̅) must be equal to slope of the CC’ line. The slope of the consumption line CC’ is the MPC whose value must always be less than unity.

Diagrammatically, this means that the (C + I̅) line must cut the 45° line from above. If (C + I̅) line cuts the 45° line from below then the value of MPC would be greater than unity and equilibrium income thus determined in this manner would be unstable.

Method # 2. Savings-Investment Approach:

Total withdrawals from and injections into the circular flow determine equilibrium national income. In a two-sector economy, withdrawal comprises only saving, while injection comprises only investment.

Equilibrium national income is determined at that point when planned saving and planned investment are equal to each other. Diagrammatically, at the intersection of the saving and investment line, equilibrium national income is determined.

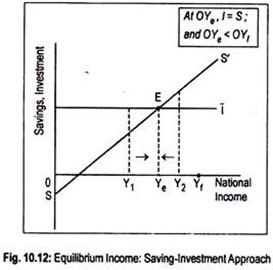
In Fig. 10.12, we measure national income on the horizontal axis and savings and investment on the vertical axis. SS’ is the planned savings curve which has a negative intercept in the sense that, at low level of income, since consumption exceeds income, savings must be negative.

As usual, I̅ is the autonomous investment line drawn parallel to the horizontal axis. As SS’ curve cuts I̅ at point E, equilibrium level of income is, thus, determined at OY_e. In other words, planned saving and planned investments are equal only at the intersection of the two curves and, thus, equilibrium income is OY_e. And this equilibrium income is a stable one.

To see whether OY_e is a stable equilibrium income, we consider OY₁ or OY₂ level of income. If the deviation from OY_e level of income gets corrected or if the equilibrium income OY_e is attained after deviation, then equilibrium is said to be a stable one.

At OY₁ level of income, investment (injection) exceeds saving (leakage). Aggregate demand must exceed aggregate output. This will result in an unplanned reduction of inventories to meet excess demand. Consequently, output will rise until planned saving and planned investment are equal. Similarly, at OY₂ level of income, since saving exceeds investment, aggregate demand falls short of aggregate supply.

Hence, an excess supply of commodities will appear leading to an unplanned accumulation of inventories. This will act as an incentive to cut back output. Output will continue to decline until point E is reached where OY_e equilibrium level of national income is determined. Thus, OY_e is a stable equilibrium.

The condition for stability is that the saving curve must be positively sloped. MPS is the slope of the saving function. To have stability the value of MPS must be positive but less than one. Remember that MPS is complementary to MPC. If MPC < 1, then MPS must be less than one since MPC + MPS = 1. Thus, the condition for stability in equilibrium income in both the approaches is the same, i.e., 0 < MPC < 1.

A Numerical Example on the Determination of Equilibrium National Income in a Two-sector Economy:

Suppose, consumption (C) is given by the consumption function

C = 5 + 0.8Y

Where, Y is income.

Assume that investment is autonomous (I̅) and is given by

I̅ = Rs. 10

It says that investment is an exogenous variable. As it is determined outside the model, investment is simply considered as given.

With this information, we want to derive the equilibrium values of income, consump­tion saving, and investment.

Solution:

Equilibrium condition is Y = C +1, where C = a + bY and I = I̅. Putting the values of C and I, we obtain

Y = a + bY + I̅

Or,Y – bY = a + I̅

or, Y (I – b) = a + I̅

.^.. Y = 1/1 – b (a + I̅)

Here, Y is the equilibrium level of income. Substituting the consumption and investment equations into equation Y, we get

Y = 1/1 – 0.8 (5 + 10) = Rs. 75

Thus, C = 5 + 0.8(75) = Rs. 65, and

S = Y – C = 75 – 65 = Rs. 10

I = Rs. 10

The above approach has an alternative approach, known as saving-investment approach.

Alternative Solution:

Equilibrium condition now is S = I

S = Y – C 

= Y – a – bY

= – a + (1 – b) Y

Assuming I̅ = I̅, the equilibrium condition becomes

– a + (1- b) Y = I̅

So the equilibrium level of income is

Y = 1/1 – b (a + I̅)

From the consumption function C = 5 + 0.8Y we find the values of -a and (1 – b). If a = 5 then (-a) = -5 and if b = .8 then (1 – b) = 0.2. Now putting these values into saving and investment equation, we get

– 5 + 0.2Y = 10

0.2Y – 5 = 10

0.2Y =10 + 5

Or, Y = 15/0.2 = Rs. 75

If Y = Rs. 75, saving must be equal to Rs. 10 since consumption is Rs. 65.

Note that since the two forms of equilibrium condition are equivalent, the level of income must be the same.