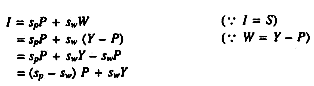Basic Kaldor’s Model (With Diagrams)

Read this article to learn about the basic Kaldor’s model in neo-classical theory of economic growth.

Introduction:

It has been seen that the original Harrod-Domar model (hereafter, mentioned as H-D Model) is rigid, light, one sector and specific with respect to three parameters.

A constant proportion of income is assumed to be saved (S_t/Y_t). The full capacity condition means a constant capital output ratio (C/O) and further the condition that on full employment the demand for labour (associated with full capacity output) must grow at the constant rate (n).

Thus, on account of constant saving-income ratio, constant capital-output ratio and constant demand for labour on full employment, the H-D model becomes too rigid to be much use. But the H-D model becomes very useful if these conditions are relaxed. The parameters (constant variables) may be allowed to vary. We may vary the supply of labour and treat it as more flexible on full employment—this has been done by Mrs. Joan Robinson and her colleagues in Cambridge.

Her ‘Golden Age Model’ is discussed further. Again, we can take a varying band of values for capital-output ratio, thereby increasing the possibility of G_w being equal to G_n. This is the position of Neo-classical models developed by R.M. Solow, T.S. Swan, J.E. Meade, Samuelson, H.G. Johanson, and others. Lastly, we may allow the saving-income ratio to vary according to the distribution of income between wages and profits (Y = W + P). This is the approach adopted by Kaldor and, therefore, we discuss his basic model first of all.

His model is based on certain assumptions:

Assumptions:

1. There are two factors of production capital and labour (K and L) and thus only two types of income profits and wages (P and W). All profits are saved and all wages are consumed.

2. There are constant returns to scale and production function remains unchanged over time. Capital and labour are complementary.

3. There is perfect competition as such the rates of wages and profits are same over different places.

4. The marginal propensity to consume of workers is greater than that of capitalists.

5. The investment-income (output) into (I/Y) is an independent variable.

6. There is a state of full employment so that total output or income (Y) is given.

7. There is an unlimited supply of labour at a constant wage in terms of wage goods.

Besides, Kaldor took certain facts as the bases of his model and as a starting point; for example, according to him, there is no recorded tendency for a falling rate of growth of productivity; there is a continued increase in the amount of capital per worker; there is a steady rate of profit on capital at least in the developed country; there is no change in the ratio of profits and wages—a rise in real wages is only in proportion to the rise in labour productivity; the capital-output ratios are steady over long periods—this implies near identity in the percentage rates of growth of production and of the capital stock; there are appreciable differences in the rate of growth of labour productivity and of total output in different sectors or economies.

Features:

The starting point of Kaldor is the belief that the income of the society is distributed between different classes, each having its own propensity to save (K = W + P). The equilibrium can be brought about only by a just and appropriate distribution of income. In other words, growth rate and income distribution are inherently connected elements. Kaldor’s model depends on these two elements and their relationships and brings forth the importance of distribution of income in the process of growth— this is one of the basic merits of Kaldor’s model.

In his model, on the one hand, the relations of distribution of income determine the given level of saving (or social saving) and, therefore, investment and economic growth rate. On the other hand, the achievement of this or definite growth rate requires a given level of investment and, therefore, of saving and hence, a corresponding distribution of income.

This is illustrated by the following system of equations:

Y = W + P ; I = S ; S = S_w + S_p,

where Y is the national income ; W—the income of labour (wages) ; P—the income of entrepreneurs (profit) ; I—investment ; S—saving ; S_w—saving from wages ; S_p—saving from profits.

But S_w = S_wW and S_p = S_pP

where S_w is the share of saving from wages ; and S_p is the share of savings from profit, substituting for S, we get:

where P/Y is the share of profit in the total income and I/Y is the investment income ratio, Now, we can easily see and appreciate Kaldor’s thesis. His thesis is that the share of profit in the total income is a function of the ratio of investment to income (I/Y).

In the above equation, it can easily be seen that an increase in the income-investment ratio (I/Y) will result in an increase in the share of profits out of total income (P/Y), as long as it is assumed that both s_w and s_p are constant and further that s_p is greater than (s_p > s_w). Thus, given the mps, of wages earners (s_w) and the mps of entrepreneurs (s_p)_} the share the profits (P) in the national income (Y), that is P/Y depends on the ratio of investment (I) to total income or output (Y), that is I/Y. In other words, P/Y is a function of

Of greater importance to us is the underlying economic rationale for Kaldor’s theorem that the share of profit in the total income (P/Y) is a function of the investment-income ratio (I/Y). Under full employment conditions an increase in investment must in real terms, bring about an increase in both the ratio of investment to income (I/Y) and also an increase in the savings income ratio (S/K). This is necessary if equilibrium at a higher level of real investment is to be obtained.

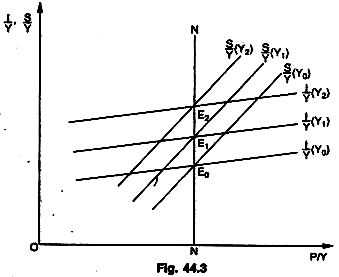
If the saving-income ratio did not rise, the result would be a continuous upward movement of the general level of prices. The heart of Kaldor’s theory lies in his demonstration “that shift in the distribution of income is essential to bring about the higher-saving income ratio, which is the necessary condition for a continued full employment equilibrium with a higher absolute level of investment in real terms. This is illustrated by the given Fig. 44.3.

In the Fig. 44.3, a direct relationship between P/Y and I/Y is assumed. The ratio of investment to income depends upon exogenous (outside) factors and is assumed as independent altogether. Since, propensities to save for the two income classes differ the mps out of profit income are more than the mps out of wage income.

Assumption of s_p > s_w, according to Kaldor, is a necessary condition for both stability in the entire system and an increase in the share of profit in income when the investment- income ratio rises. Given the full employment income Y₀, the investment-income ratio and the saving- income ratio (I/Y) and (S/Y) are I/Y (Y0) and S/Y (Y₀) and the system is in equilibrium with the profit income ratio fixed by the vertical line AW.

If there is an increase in income, both S/Y and I/Y function shift by such magnitudes that they assume the position S/Y (Y₁) and I/Y (Y₁). The equilibrium profit share will remain constant as measured by the line NN. Had there been a shift in the I/Y with S/Y function at S/Y (Y₀), there would have been an inflationary price movement.

But an increase in P/Y, assuming that S_p > S_w, pushes up the S/Y function to ensure equilibrium at full employment. If this smooth movement between I/Y with S/Y persists the system will sustain itself at full employment and the equilibrium share of profit to income will remain constant. The underlying idea is that with fixed level of real income (assumption of full employment), the only way in which it is possible to bring about an increase in S/Y for the entire economy is either through a rise in the propensity to save itself, which has been ruled out by Kaldor through his assumption that S_p and S_w are constant, or through a shift in the distribution of real income from low saving groups to the high saving groups.

The mechanism which brings about the redistribution of income in favour of the profit share whenever there is a rise in the investment-income ratio is essentially that of the price level. The increase in investment expenditure under full employment conditions, leads initially to a general rise in prices. But wages cannot rise as fast and as much as the rise in prices.

The failure of money wages to keep pace with the rise in prices will reduce real income of wage earners and it will increase the profit margins of entrepreneurs. Since the mps of the latter group is, on the average higher than that of wage earners, the inflation induced shifts in the distribution of real income in favour of profits will increase the overall level of real saving in the economy.

This process will continue until the saving- income ratio (S/Y) is once again in equilibrium with the investment income ratio (I/Y). Thus, it is quite clear that the assumption of s_p > s_w is of crucial importance in the Kaldor’s model. In the absence of this assumption, the real S/Y will not rise irrespective of any change in the distribution of income. Consequently, the system may remain unstable.

Kaldor and Harrod:

We find, that s_p > s_w is the basic equilibrium and stability condition. If s_p < s_w, there will be a fall in prices and cumulative decline in demand, price and income. Similarly, if s_p > s_w, there will be a rise in prices, cumulative rise in demand and income. The degree of stability of the system is dependent on the difference between the marginal propensities to save. If the difference between the two propensities (s_p and s_w,) is small, the coefficient 1/ s_p –s_w will be large with the result that small changes in the investment-income ratio (I/Y) will lead to relatively large changes in income distribution (P/Y) and vice-versa.

Kaldor, in his writing or model, tries to find these causes (of this stability or instability) in the purely techno- economic regularities or irregularities of growth. To simplify the reasoning, he assumes that the mps of wage earners (s_w) is zero.

In these circumstances, the equation given above becomes:

According to Harrod’s model, the rate of accumulation (I/Y) is determined by the growth rate and the capital output ratio, that is

The economic meaning of this equation is that the share of profit in income is determined by the share of savings out of profit income (s_p), the growth rate (G) and the capital output ratio (C_r). If the first two indicators remain constant, the stability of the share of profit in income (P/Y) will then be determined by the stability of capital coefficient (C_r). To explain and to substantiate this stability, Kaldor introduced his famous technical progress function. Thus, under Kaldor’s model, the share of profit, the rate of profit—which establishes S and I identity, assisted by technical progress function,¹ provides the mechanism of growth, stability and dynamics.

Critical Evaluation:

The basic features or novelties of Kaldor’s model may be summed up as follows:

(a) Its great merit lies in the development of the concept of technical progress function and the belief that the technical progress acts as the main engine of growth. Technical progress function under Kaldor’s model replaces the usual production function. According to him, the basic functional relationship is not the production function expressing output per man as an increasing function of capital per man—but a technical progress function expressing the rate of increase in output per man as an increasing function of the rate of increase of investment.

(b) Another great merit of Kaldor’s model lies in the views—that the inducement to invest does not depend on MEC or interest rate comparisons ; the rejection of long-run underemployment equilibrium; the introduction of a distribution mechanism into Harrod’s model. Kaldor’s model though essentially based on Keynesian concepts and Harrodian dynamic approach differs from them in a number of ways. Kaldor believes that economic growth and its process are based on the interdependence of the fundamental variables like savings, investment, productivity, etc.

In Kaldor’s opinion a dynamic process of growth should not be presented and cannot be understood with the help of certain constants (like constant S_t/V_t or C/O ratio under Harrod’s model) but in terms of the basic functional relationships. The basic fundamental relationships among the fraction of income saved, the fraction of income invested and the rate g increase of productivity per man, determine the outcome of the dynamic process.

Limitations of the Model:

(a) Since Kaldor seeks to relate the functional distribution of income directly to variables that are of crucial importance in the determination of the level of income and employment, his analysis is rightly described as an aggregate or macroeconomic theory of income distribution. But his analysis is severely restricted by its underlying assumptions.

The theory does not tell us how the distribution of income in a functional sense will be affected by changes in real income below the full employment level, though it does tell that any attempt to increase capacity and full employment is reached, will bring about a relative increase in the non-wage share in the total income. In this sense, Kaldor’s model has a distinct classical flavour, even though his framework is that of modern employment theory.

(b) It is on account of its restrictive assumptions that Kaldor’s model is not easily generalised for more than two classes. His assumption of invariable shares of income saved (s_p and s_w)—is much too rigid. Empirical analysis shows that these shares tend to change over time depending on income growth and other factors. While Kaldor himself remarks on the excessively generalised nature of his conception, one must say that its fundamental methodological flow amounts to more than that.

It is an attempt to fit into the rigid framework of purely technological change the whole complexity of socio-economic changes, which characterise the growth of free competitive capitalism into monopoly and state monopoly capitalism—changes which had/have an effect on the distribution of the national income (in a manner postulated by Kaldor according to his assumptions).

(c) Moreover, Kaldor’s abstract model takes no account at all of the vast unproductive expenditure which burden modern capitalist society, especially government military spending. The introduction into his model of state income with a corresponding ‘propensity to save’ could upon up a source of growth and rising rates of accumulation other than the wage earner’s income.

(d) Kaldor’s model, in its present state cannot be accepted either as a model of growth or as a model of macro-distribution. His model depends upon a unique profit rate, which has the needed value to produce or ensure steady—state growth—but he doesn’t tell or show, how this unique rate of profit is determined ? This, in fact, is a great shortcoming of his model and the line of thought has to be developed further to make it more fruitful; the aim being to develop a general equilibrium model of growth. The model, therefore, needs to be supplemented by a theory of income distribution.

(e) His distribution mechanism through what has been described above as ‘Kaldor Effect’ has also been criticised. A continuing rise in prices has different results like over spending, wage inflation, wage-price spiral and these consequences determine income distribution. His model attributes all profits to capitalists, thereby implying that workers savings are transferred as a gift to capitalists, this is obviously absurd—for under these conditions, no individual will save at all. That is why it is remarked whether Kaldor’s model of distribution does provide a satisfactory alternative or does it involve a jump from the frying pan into the fire?

That is why Prof. J.E. Meade remarked that—can it be really maintained that when Kaldor effect takes place and prices and selling prospect are improving—wages will remain unchanged ? Will not the entrepreneurs bid up the wage rate against each other to employ labour under the impact of Kaldor effect? How else can one explain the notorious phenomenon of wage drift? Will not the authorities take steps to correct or offset the initial inflation of investment? Mr. Kaldor’s theory of distribution is more appropriate for explaining short- run inflation than long-run growth.

(f) Kaldor’s Model fails to take into consideration the impact of redistribution of income on human capital. His theory lays emphasis on physical capital. McCormik remarks, “the failure of the theory to incorporate human capital leaves the theory too simple to explain the complexities of the real world.” With an increase in I/Y, the share of profit (P/Y) will increase and the share of labour will fall, deteriorating human capital—which in turn, will bring a reduction in income output.