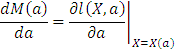Envelope Theorem for Constrained Optimization | Production | Economics

The Envelope theorem is explained in terms of Shepherd’s Lemma. In this case, we can apply a version of the envelope theorem. 

Such theorem is appropriate for following case:

Envelope theorem is a general parameterized constrained maximization problem of the form

Such function is explained as h(x₁, x₂ a) = 0. In the case of the cost function, the function is written as

The above function explains a price. The Langrangian for this problem can be written as

For the above function, we need to take the first order condition.

It is as follows:

Similarly

Above condition determine the optimal choice function (x₁(a), x₂(a)).

It determines the maximum value function:

The envelope theorem gives us a formula for the derivative of the value function. It is with respect to parameter in the maximization problem. The formula is given as

The interpretation of partial derivatives needs special care. They are the derivatives of g and h with respect to a holding X₁ and x₂ They are the fixed at their optimal values. The proof of the envelope theorem is straight forward and it is calculated in the following equation.

Differentiating the identity above to get:

And if we substitute from the first order condition of equation 51 then we get the following equation:

From the above equation, we can observe that the optimal choice function must identically satisfy the constraints.

It is as follows:

Suppose, we differentiating this identity with respect to a, then we have,

Now we substitute (54) into (53) to get the following equation:

Above equation is required for further interpretation of result. Such results can be used for the cost minimization problem. In the cost minimization problem, the parameter can be chosen to be the factor price w_i. The optimal value function M (a) is a cost function. It is presented as c (w, y).

The envelope theorem explains that:

Above function is simply a Shephard’s Lemma. The proof is given as follows. Let us assume that x₁(w, y). It is the firm’s conditional factor demand for input i. suppose the cost function is differentiable at (w, y) and wi > 0 for i = 1….n.

It can be derived as follows:

Proof:

Let us assume that x* is a cost minimizing bundle. It produces y at price w*. We define the function as

In the above equation, c (w, y) shows that, it is easy way to produce y. Such function is always negative.

At w = w*, g (w*) = 0. Since this is a maximum value of g (w) and it is already given. Its derivative must vanish.

Hence the cost minimizing input vector is given by the vector of derivatives. It is a cost function with respect to the prices. We can explain the Shepherd’s Lemma after adding the above proof. The Shepherd’s Lemma is an important result in microeconomics. It has the application in consumer choice preference and theory of firm. It helps to understand how consumer chooses commodities in their consumption bundle. The lemma states that if IC of expenditure or cost function is convex, then the cost minimizing point of a given good (i) with the price p_i is unique.

The ideal case is that the consumer will buy a unique amount of each item. Such item minimizes the price for obtaining a certain level of utility given the price of goods in the market. Such theory and proof was named after Ronald Shepherd. He presented research paper in 1953. In his paper, he gave a proof using the distance formula. But such formula was already used by John Hicks (1939) and Paul Samuelson (1947) in their model. The lemma gives a precise formulation for the demand of each good in market with respect to that level of utility and prices.

The derivative of the expenditure function (e (p, u)) with respect to price is given as follows:

Where,

hi (u, p) is the Hicksian demand for good i.

E (p, w) shows an expenditure function and both function are in terms of a price (a vector p) and utility u.

Although Shepherd’s original proof is used in the distance formula. It is a proof of the Shepherd’s Lemma in the envelope theorem.