The Laws of Returns to Scale: IRS, CRS and DRS

Let us study about the the Laws of Returns to Scale. After reading this article you will learn about: 1. Introduction to the Laws of Returns to Scale 2. Explanation of IRS 3. Explanation of CRS 4. Explanation of DRS.

Introduction to the Laws of Returns to Scale:

If a firm changes all its inputs in the same proportion then we can say that there occurs a change in the scale of production.

The study of changes in output following a change in the scale of production results in “laws of returns to scale” which has to be distinguished from the “laws of returns to a variable input” obtainable in the short run. A firm can change its scale of operation only if a sufficiently long time is allowed. Thus, the “laws of returns to scale” form the subject- matter of long run production function.

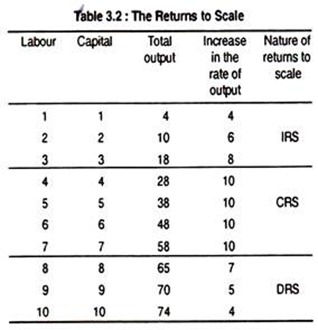
We will now describe returns to scale with the help of a table and graph. For simplicity’s sake, we assume that there are two inputs— labour and capital. Table 3.2 shows how output changes when these inputs are used in certain fixed ratio.

 

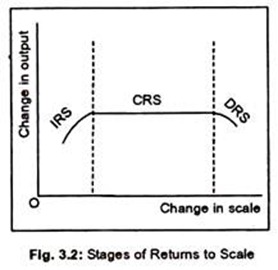
The table suggests that the firm initially experiences IRS when it expands output, after then CRS, and, finally, DRS. Fig. 3.2 also illustrates how change in scale occurs as output changes. Now we will trace out causes behind each stage of returns to scale.

If all inputs are increased by the same proportion—keeping factor proportions unaltered—then there occurs an increase in scale. The percentage increase in output due to one per cent increase in all inputs determines the returns to scale.

Suppose, one-output three-variable input production function is given by:

Q = f (L, B, K)

Now, if all these inputs are exactly doubled then output may be doubled. If so, then returns to scale are said to be constant. Suppose all L, B, K inputs are increased by 3, then output will also increase by 3, i.e.,

3Q = f (3L, 3B, 3K)

If doubling or trebling of inputs cause output to increase by more than 3, then we have increasing returns to scale. And, returns to scale are said to be decreasing when doubling of inputs results in turning out output less than that.

As firms increase the scale of production, initially the increase in output is subject to increasing returns, then constant returns and, finally, diminishing returns to scale. Initially, if we increase all the inputs by one percentage point, output will increase by more than one percent. Then we have the stage of increasing returns to scale (IRS).

Now, if the rate of increase in output remains constant following a one per cent increase in input, then we have the stage of constant returns to scale (CRS). Later on, the firm experiences diminishing returns to scale (DRS). The firm experiences even diminishing returns to scale (DRS) as scale of production rises.

If:

(i) Percentage change in TC < percentage change in Q

(ii) Percentage change in TC = percentage change in Q

(iii) Percentage change in TC > percentage change in Q

Then:

(i) Economies of scale (IRS) [Decreasing LAC]

(ii) No economies or diseconomies of scale (CRS) [Constant LAC]

(iii) Diseconomies of scale (DRS) [Increasing LAC]

Explanation of IRS:

In the first place, the size of a firm is the most proximate cause of the emergence of IRS. As the size of a firm increases, returns to scale becomes increasing. One large-sized factory seems to be more efficient than two small- sized firms.

If the diameter of a pipe is doubled, flow from it will be more than double. Similarly, a double-decker bus can carry twice the number of passengers as does a single-decker bus at the same total cost. Thus, the economy of increased dimensions argues for IRS.

Secondly, the technical and/or managerial indivisibility also explain IRS. Some of the factors are large and lumpy since these do not come in small units. For example, one cannot install half a blast furnace! A large- sized firm can use these lumpy and indivisible factors most efficiently. Lumpiness is the most important source of economies of large scale production.

Thirdly, indivisibility of inputs and specialization are interrelated. With the increase in size, this is also called increased division of labour that follows from Adam Smith’s famous pin-factory case. The degree of specialization tends to rise. As a result, efficiency and productivity of an input will rise.

Due to the interplay of these factors, large scale firms enjoy economies of production— both internal and external. Economies of large scale production, therefore, lie behind the existence of IRS.

Explanation of CRS:

It is true that IRS does not last long. Thus returns to scale must not be rising the only. At best, it can be constant. CRS states that if all inputs are doubled, output will also be doubled. But, it is argued that the phenomenon of CRS is a very temporary one.

If all inputs are doubled then what is there to prevent output from being doubled? There are two reasons behind this situation. Scarcity of some inputs and indivisibility of some inputs are the two causes behind DRS, rather than CRS.

So, we can oppositely argue that if these two elements do not come out, then one would have obtained CRS. Remember that, under CRS, economies and diseconomies of scale cancel each other and, as a result, long rim average cost becomes constant.

Explanation of DRS:

DRS is supposed to set in once the firm oversteps the limit of expansion. Continuous increase in all inputs and, hence, expansion in the size of a firm beyond a certain point lead to the emergence of DRS. One of the reasons behind the occurrence of DRS is the managerial inefficiency since there are limits to the efficient functioning of management.

As the size of the firm increases, inefficiency tends to rise and diseconomies of large scale production become stronger than the economies of scale.

Again, DRS may appear due to the exhaustion of fixed natural resources or physical constraints of inputs. There is no guarantee that the coal production will be doubled if the size of coal mine is doubled because the stock of coal on the earth is not plentiful in amount. This will surely raise cost of production.