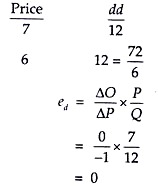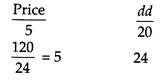Consumer Equilibrium and Demand (Questions)

Read this article to learn about the top frequently asked questions on Consumer Equilibrium and Demand.

Q.1. Starting from the point of consumer equilibrium, suppose MU of a rupee increases. Tell how is it going to make an impact on quantity demanded?

Ans. i. If MU of a rupee increases, it will disturb the condition of consumer equilibrium.

ii. In order to get back the condition of consumer equilibrium, MU of a product has to be increased.

ADVERTISEMENTS:

iii. As per law of diminishing marginal utility, more you consume additional utility from additional commodity keeps on becoming less.

iv. Therefore additional quantity demanded should be reduced in order to increase MU of a product.

Q.2. A female dentist charges Rs 300 for dental cleaning with that she generates a revenue of Rs 30,000. She increased the charges to Rs 350 but with this she lost her customers. Still she is generating a revenue of Rs 33,500. Find E_D with total outlay method.

Ans.

With the rise in price of her dental cleaning, her revenue / patients’ expenditure is increasing. As per total outlay method, if there is a direct relationship between price & expenditure then E_D < 1 (less than unity) & therefore inelastic.

Q.3. Suppose there are two consumers in the market for a good and their demand functions are as follows:

d₁(p) = 20-p for any price less than or equal to 20 and

ADVERTISEMENTS:

d₁ (p) = 0 at any price greater than 20.

d₁(p) = 30 – 2p for any price less than or equal to 15 and

d₂ (p) = 0 at any price greater than 15

Find out the market demand function.

Ans. d ₁(p) = 20-p P < 20

d₁ (p) = 0 p > 20 -d) …(1)

d₁(p) = 30-2p P < 15

d₁(p) = 0 p > 15 -(2) …(2)

For market demand function (dm)

ADVERTISEMENTS:

On adding equation (1) and (2)

dm (p) = 50 – 3p for P ≤ 50/3

dm (p) = 20-p for p ≤ 20.

dm (p) = 0 for p > 20 Q.4.

ADVERTISEMENTS:

Q.4. Suppose there are 20 consumers for a good and they have identical demand functions:

d (p) = 10 – 3p for any price less than or equal to ≤ 10/3 and d₁(p)= 0 at any price greater than 10/3

ADVERTISEMENTS:

What is the market demand function?

Ans. d (p) = 10-3p for p ≤10/3

d₁ (p) = 0 for p > 10/3

No. of consumers = 20

Market demand function dm (p) can be obtained by multiplying individual demand function by 20 since there are 20 consumers having identical demand function.

Therefore market demand function is:

ADVERTISEMENTS:

dm (p) = (10-3p)(20) for p ≤ 10/3

dm (p) = 200-60p

dm (p) = 0 for 10 > 10/3

Q.5. Suppose the price elasticity of demand for a good is -0.2. If there is a 5% increase in the price of the good, by what percentage will the demand for the good go down?

Ans. E_d = -0.2

And % increase in price = 5% are given

ADVERTISEMENTS:

E_d= % Change in Quantity Demanded / % Change in Price

-0.2% Change in Quantity Demanded

% change in quantity demanded = – 0.2 x 5

= (-)1

Q.6. Suppose the price elasticity of demand for a good is 0.2. How will the expenditure on the good be affected if there is a 10% increase in the price of the good?

Ans. The elasticity of demand in the given case is 0.2. It means the demand is inelastic since E_d is less than one. As per total outlay method, in case of an inelastic demand there is direct relation between price & expenditure. Since price is increasing, in this question, as a result the expenditure on the commodity will rise.

ADVERTISEMENTS:

Q.7. A consumer wants to consume two goods X & Y. The prices of X and Y goods are Rs 4 and Rs 5 respectively. The consumer’s income is Rs 20.

(i) Write down the equation of the budget line.

(ii) How much of good X can the consumer purchase if she spends her entire income on that good?

(iii) How much of good Y can she consume if she spends her entire income on that good?

(iv) What is the slope of the budget line?

Ans. (i) Let X and Y be good 1 and good 2.

ADVERTISEMENTS:

Price of X (P_X)= Rs 4 (Given) Price of Y(P_x) = Rs5 (Given)

Income of the consumer (M) = Rs 20

Equation of the budget line = P_x X + P_Y= M

4X + 5Y = 20

(ii) Income = Rs 20

Price of X = Rs 4 per unit

Quantity of X which can be bought = Rs 20 / Rs 4

= 5 units.

(iii) Income = Rs 20

Price of Y = Rs 5 per unit.

Quantity of Y that can be bought = Rs 20/Rs 5

= 4 units.

(iv) Slope of the Budget Line = Price of X(P_x) / Price of Y(P_x)

Q.8. Suppose a consumer can afford to buy 6 units of good 1 and 8 units of good 2 if she spends her entire income. The prices of the two goods are Rs 6 and Rs 8 respectively. How much is the consumer’s income?

Ans. Let good 1 be X

And good 2 be Y

Now, Price of X (P_x) = Rs 6

And Price of Y (P_y) = Rs 8

Quantity Purchased of X = 6 units (given)

Quantity Purchased of Y = 8 units (given)

Budget Equation = P_x + P_y.Y = M

Where P_x = Price of X and P_y = Price of Y

X = Quantity purchased of X

Y = Quantity purchased of Y

M = Money Income

Putting the values, we get

M = Rs 6.(6) + Rs 8.(8)

= 36 + 64

Answer: Money Income = Rs 100.

Q 9 Suppose a consumer wants to consume two goods which are available only in integer units. The two goods are equally priced at Rs 10 and the consumer’s income is Rs 40.

(i) Write down all the bundles that are available to the consumer.

(ii) Among the bundles that are available to the consumer, identify those which cost her exactly Rs 40.

Ans. Price of X (P_x) = Rs 10 (given)

Price of Y(P_y) = Rs 10 (given)

Money income = Rs 40 (given)

(i) Now, various bundles available to the consumer are:

(0,1) (1,0) (2,0) (3,0) (4,0)

(0,2) (1,1) (2,1) (3,1)

(0,3) (1,2) (2,2)

(0,4) (1,3)

(ii) And the bundles costing exactly Rs 40 to the consumer are:

(4, 0) (3,1) (2,2) (1,3) and (0,4)

All the rest will cost less than Rs 40.

Q.10. When price of a good is per unit a consumer buys 12 units. When price falls to Rs 6 per unit he spends Rs 72 on the good. Calculate price elasticity of demand by using the percentage method. Comment on the likely shape of demand curve based on this measure of elasticity.

Ans.

The demand curve is parallel to the V-axis.

Q.11. A consumer buys 10 units of good at a price of Rs 9 per unit. At price of Rs 10 per unit he buys 9 units. What is price elasticity of demand? Use expenditure approach. Comment on the likely shape of demand curve on the basis of this measure of elasticity.

Ans.

Since with change in price total expenditure is unchanged, elasticity is equal to -1. Demand curve is rectangular hyperbola.

Q.12. A consumer buys 20 units of a good at a price of Rs 5 per unit. He incurs an expenditure of Rs 120 when he buys 24 units. Calculate price elasticity of demand using the percentage method. Content upon the likely shape of demand curve based on this information.

Ans.

Q.13. A consumer buys 10 units of a commodity at a price of Rs 10 per unit. He incurs an expenditure of Rs 200 on buying 20 units. Calculate price elasticity of demand by the percentage method. Comment upon the shape of demand curve based on this information.

Ans.

Q.14. A consumer buys 14 units of a good at a price of Rs 8 per unit. At price of 7 per unit he spends Rs 98 on the good. Calculate price elasticity of demand by the percentage method. Comment upon the shape of demand curve based on this information.

Ans.

Q.15. A consumer buys 8 units of a good at a price of Rs 7 per unit. When price rises to Rs 8 per unit he buys 7 units. Calculate price elasticity of demand through the expenditure approach. Comment upon the shape of demand curve based on this information.

Ans.

Since with change in price total expenditure is unchanged, price elasticity is – 1 Demand curve is rectangular hyperbola.

Q.16. A consumer consumes only two goods X and Y. At a consumption level of these two goods, he finds that the ratio of marginal utility of price in case of X is higher than in case of Y. Explain the reaction of the consumer.

Ans. It means that at some consumption level

MU_x /P_x > MU_x/P_y

In this case the consumer is getting more marginal utility per rupee in case of good X as compared to Y. Therefore, he will buy more of X and less of Y. This will lead to fall in MU_x and rise in MU_y. The consumer will continue to buy more of X till MU_y P_x becomes equal to MU_y/P_Y.

Q.17. A consumer consumes only two goods X and Y. At a certain consumption level of these goods, he finds that the ratio of marginal utility to price in case of X is lower that in case of Y. Explain the reaction of the consumer.

Ans. It means that at some consumption level MU_x /P_x is less than MU_y/P_y. As such the consumer is getting less MU_x per rupee as compared to MU_y. Therefore, he will buy more of Y and less of X. This will lead to fall in MU_y and rise in MU_x till MU_x/P_x becomes equal to MU_y/P_y.