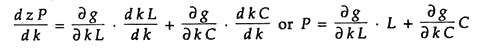Euler’s Theorem of Distribution (With Diagram) | Firm

In this article we will discuss about Euler’s theorem of distribution.

According to marginal productivity theory, every input is paid the value of its marginal product. This means that the entire product will always be handed out to those who work on it. In other words, the sum of the marginal products add up exactly to the total output. There is thus neither a surplus nor a deficit left at the end.

This proposition can be proved by using Euler’s Theorem. It suggests that if a production function involves constant returns to scale (i.e., the linear homogeneous production function), the sum of the marginal products will actually add up to the total product.

This can be proved by the total differentiation theorem. Now, if we have the function z = f(x, y) and that if, in turn, x and y are both functions of some variable t, i.e., x = F(t) and y = G(t), then

So the effect of a change in t on z is composed of two parts: the part which is transmitted via the effect of t on x and the part which is transmitted through y. Thus, the latter is represented by the expression (∂f/∂y) (∂y/∂t).

Here (dy/dt) shows the change in y produced by the increment in t and (∂f/∂y) is the resulting change in z produced by each unit of this change in y.

It follows from a linear homogenous production function

P = g (L, C), where we have, for any k,

kP = g(kL, kC)

If we now take the total derivative of kP with respect of k [i.e., setting kP=z, kL=h, kC=y, and k=t in our formula for (dz/dt)] we get

Since this result holds for any value of k it is must also be valid for k = 1 so that

P = ∂g/∂L L + ∂g/∂C C

This is Euler’s Theorem for the linear homogenous production function P = g (L, C). The proof can be extended to cover any number of inputs. Since ∂g/∂L is the marginal product of labour and ∂g/∂C is the marginal product of capital, the equation states that the marginal product of labour multiplied by the number of labourers (each of whom is paid this amount) plus the corresponding total payment to capital exactly equals the total product, P.

According to Paul Samuelson, whether there will any profits or surplus for the entrepreneur depends or market conditions. If, for instance, we consider a situation of perfect competition, in the long-run prices of inputs and outputs will settle towards levels at which there is nothing left over for payment to the entrepreneur in excess of his managerial wages and interest on his capital. There will be a profit in excess of this amount only if there is monopoly.